On the Stability of the Gliese 876 System of Planets and the Importance of the Inner Planet - by Ricky Leon Murphy:

Back to Exoplanets

This is one of the major projects for the Swinburne Astronomy Online Master's Degree Program. This is actually part two of my two-part exoplanet subject. While both papers are not directly related to each other, it does offer a look at both the amateur and professional involvement in exoplanet research.

For part one, Exoplanet Detection, click the link.

Click for poster

Abstract
Introduction
Gliese876 and the System of Planets
Methods for Determining Mass Using High-Resolution Spectroscopy
Orbital Dynamics
The N-Body Problem and the SWIFT Simulator
Solar System Simulations
Gliese 876 Simulations
Discussion
Summary
References

Abstract: A third planet with a mass of 0.023 M_J was found orbiting the star Gliese 876. The initial two body system was found to have a perfect orbital resonance of 2/1. This paper will demonstrate the orbital stability to maintain this ratio is highly dependant on the presence of the small, inner planet. In addition, any change in orbital eccentricity of the inner planet as well as initial planetary set-up will also severely alter the orbital dynamics of the remaining two bodies. In addition to this demonstration, methods of detecting the planetary system will be covered as well as the theories and techniques used to plot the orbits of these bodies.

Back to Top

1 - Introduction

A system of planets was found to orbit the star Gliese 876. This is very unique as this is a class M star – the initial target of exoplanet searches were G and K type stars.  A class M star burns at a lower temperature than our Sun, and is a third of the mass of our Sun. The Astronomy Picture of the Day has a fairly un-impressive image of this star. Equally unique is its distance, only 15 light-years away – Gliese 876 is one of a handful of stars that is close to Earth.

This relatively un-impressive star is now host to three planets, two of which orbit in a harmonious 2/1 resonance. The outer two planets are what have been coined as “hot-Jupiters.” That is, giant Jupiter sized planets that reside close to their host, and are therefore hotter in temperature. The inner planet is one of the least massive planets ever detected orbiting another star – at nearly 7.5 M_E. This is an impressive feat as the method used to detect these stars is high resolution spectroscopy from telescopes here on Earth.

This paper will look at the stability of the current Gliese 867 system. The works of Innanen et al. [R9] speculate as to the instability of our own Solar System minus the Earth-Moon dynamic as well as without the inner Solar System. I will use this example to determine the importance of the inner planet to the orbital stability of the outer two planets of the Gliese 876 system.

Section 1.2 will cover the history of planetary detection of Gliese 876. Section 2 will discuss the methods used to determine the masses of the Gliese 876 members through high-resolution spectroscopy. Section 3.1 will discuss the mathematics involved in determining orbital dynamics and Section 3.2 will cover the software used to implement these parameters and discuss the method used to derive our results. Section 4 will cover the actual simulation of our Solar System and the effects of the inner planets while Section 5 will cover the simulations of the Gliese 876 system. Following these sections will be a discussion as well as a summary and opinion of results.

Back to Top

1.2 – Gliese 876 and the System of Planets

The exoplanetary detection team led by Professor Geoffrey Marcy is responsible for the majority of detected exoplanetary systems. This team uses high-resolution spectroscopy from both the Lick and Keck observatories. While the main focus of exoplanetary searches have been focused on main-sequence G and K type stars, nearby M class stars have been included in the search. Interestingly, the M class main-sequence stars make up almost 70% of all stars in our galaxy [R16].

Gliese 876 is a class M4 star with a visual magnitude of 10.1. Its luminosity is 0.0124 L_Sun and mass of 0.32 M_Sun. Using high-resolution Echelle type spectrometers – the Hamilton and HIRES – a 2.1 M_J mass planet was discovered orbiting Gliese 876 at a distance of only 0.2 AU. Using Keplerian mechanics, the planet was found to orbit the planet every 61 days [R16]. This data was reported to the Astronomical Journal on October 1, 1998.

Continual simulation by the Marcy team of Gliese 876 revealed certain issues with simulated data and observed results. After adding Fourier components to the Keplerian mathematics, it was discovered that a second body was responsible for explaining the simulated results. Since spectroscopic data had continually been collected on this star since the 1998 report, the Marcy team was able to compare the new simulation results with current observed data. On July 20, 2001, a paper was published announcing Gliese 876 was host to two bodies, the inner planet with a mass of 0.56 M_J and the outer planet with a mass of 1.89 M_J. What’s more, these two planets are in a 2/1 orbital resonance which is why the inner planet was undetected initially [R17].

Since the discovery, a host of papers have been written about the simulation results of Gliese 876 in regards to its “perfect” 2/1 resonance [R2][R10][R11][R13][R14][R15][R20][R21]. In addition, simulations of this system are stable to a million years. Instability in the system did occur with changes in eccentricity – which seems to play a vital role in resonance – as well as changes in the initial position of the planets prior to running the simulation [R20]. Ji et al. [R10] states that orbital stability is very sensitive to the simulations initial setup – that is the initial location of the two planets. In addition, the 2/1 resonance seems to occur and high inclinations and is vital to overall system stability.

Laughlin et al. [R15] and Zhou et al. [R24] take the orbital simulations of Gliese 876 to a different level in that test particles are inserted between Gliese 876 and the inner planet. The results seem to support that a third body could exist in this region. A pre-print paper [R22] was release just a few months ago the reveal a third body discovered between Gliese 876 and the now middle planet. Labeled Gliese 876d, this planet is a small ~7.5 M_E body that orbits the host star at only 1.94 days. This is the least massive extrasolar planet detected to date.

Table 1: Gliese876 System of Planets – 1998 [R16]:

Table 2: Gliese 876 System of Planets – 2001 [R17]: 

Table 3: Gliese 876 System of Planets – 2005 [R22]: 

Back to Top

2 – Methods for Determining Mass Using High-Resolution Spectroscopy

At this point of the paper, it is important to reveal the techniques used to determine the mass of these (and other) extra-solar planets. While mathematics and computer simulation are vital in this regard, there has to be a starting point. Some of the introductory work in introducing the methods used to detect extra-solar planets is found in a project paper I wrote for one of my previous SAO classes, found here: http://astronomyonline.org/Exoplanets/Exoplanets.asp.

To summarize, extra-solar planets are detected by one of two main methods:

• Planetary transit
• Radial velocity

The radial velocity method – the method used to detect the bodies orbiting Gliese 876 – makes use of a special type of spectrometer called an Echelle. This spectrometer extends the focal length of the light while using a very precise diffraction grating and high resolution CCD camera. This type of spectrometer is capable of detecting radial velocity changes to 15 m/s. Further improvements were possible with the introduction of an iodine gas over the incoming spectra to serve as a composite spectrum. This technique improved radial velocity changes to an astounding 3 m/s.

Detecting the mass of a planet orbiting a star using spectroscopy is not a very accurate one, but does give Astronomers a starting point to use in follow-up computer simulations to determine the orbital parameters. Generally the only values that are given directly will be the mass of the host star and an orbital velocity. Using a modified Doppler formula will allow us to determine the mass:

                                 (1)[R19]

The mass of the planet is given in m_psin i since we usually do not know the inclination. The value M is the mass of the host star, P is the Doppler period and K is the semi-amplitude of the Doppler shift. To solve for K:

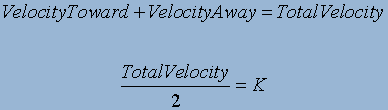                               (2).

If we know the orbital period of the planet from radial velocity measurements and we use equation 1 to determine the mass, we can determine the semi-major axis by using Keplerian math:

                                                          (3).

It should be noted that P should be converted to seconds and mass should be converted to kilograms.

Using observational data, high-resolution spectroscopy and Keplerian mathematics, it is possible to determine the mass of the star, mass of the planet, the orbital period, and the semi-major axis. These values are enough to insert into a simulation program to determine the other important orbital characteristics – covered below.

Back to Top

3.1 – Orbital Dynamics

Orbital dynamics is a set of parameters that accurately represent a system of planets. There are two variations of orbital dynamics: Keplerian and Cartesian. Keplerian coordinates are a coordinate system based on angles while the Cartesian coordinate system is based on points and vectors. Both are a means to an end, their method of arrival is just a bit different. The challenge of this paper is the literature used for reference uses Keplerian coordinates while the simulation software used for this project (SWIFT) uses Cartesian coordinates.

Keplerian Coordinates:

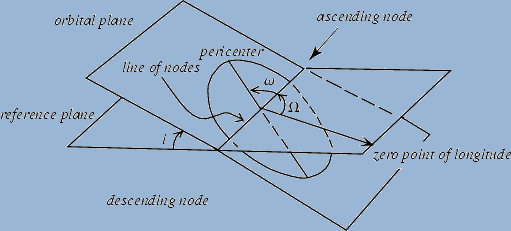
Figure 1

Figure 1 demonstrates the Keplerian coordinate system. One of the issues with this project is the true anomaly. The orbital elements that define the Gliese 876 system in the literature use a Mean Anomaly – which is the average position of the planet over time. However, since the computer simulation used for this project uses Cartesian coordinates, it is required to convert the Mean Anomaly to the True Anomaly so a Keplerian to Cartesian conversion can take place. 

In order to make this happen, the eccentric anomaly must be found. We are lucky in this regard as we have the value for the Mean Anomaly given: 

                                                (4)[R6].

Where M is the mean anomaly, E is the eccentric anomaly and e is the eccentricity.

Once the eccentric anomaly (E) is found, we can use the following equation to find the true anomaly:

                                            (5)[R6].

Where v is the true anomaly, e is the eccentricity and E is the eccentric anomaly.

Now that we have all the values defined for the Keplerian coordinate system, we can look at the Cartesian coordinate system – which is a system of coordinates in a three-dimensional space.

Cartesian Coordinates:

Both coordinate system offer both position and overall orbital predictability – that is we can tell where an object will be as well – or at least hope to be. These systems of coordinates do not take into account the gravitational interactions between bodies.

There is a relation between both coordinate systems.

Relation between Keplerian and Cartesian Coordinates:

Back to Top

3.2 – The N-Body Problem and the SWIFT Simulator

In terms of the mathematics involved in solving for the orbit of planets, a two-body system is the only system that can be solved completely [R4]. The reason is that only two bodies are interacting with each other and the forces are equal.

The following equations outline the 2-body problem:

 and                                     (6)[R1].

Since the only forces acting on each other are equal, the 2-body problem is completely solvable, without any need of mathematical variations. 

The 2-body problem above does not include other factors required for simulating the two body system, but I will introduce them later. 

An N-body system where N > 2 is not so easily solvable; in fact there is no complete solution to the N > 2-body problem. The issue arises with the mass interacting with each other – they are not equal: 

 ,  and              (7)[R4];

where F_1-2 is the force between bodies 1 and 2, F_1-3 is the force between bodies 1 and 3, and so on. The above example shows the forces of three bodies, but with additional bodies will add to the complication of the system.

The forces of the N-body problem can be summed: 

                                                           (8),

where  n = i and j = n-1; n is the body. The value for e is called the “softening” parameter and is programmed into the SWIFT code. This allows a computer to compensate if the distance between two bodies gets too close.

In addition to the forces exerted by each body, the tidal forces must also be considered. A large central body – in our case, a star – exerts a centrifugal force on the orbiting body. The orbit of this body while feeling the force is called a Hill Radius:

                                                               (9),

where μ is the reduced mass of the two bodies: 

                                                            (10).

In addition to calculating the forces, calculation of time evolution and velocity is also required. For position: 

                                                               (11),

where r_f is the final position, r_i is the initial position, v_i is the initial velocity and Δt is the change in time. For velocity: 

                                                             (12),

where v_f is the final velocity, v_i is the initial velocity, F is the force exerted (equation 6) and Δt is the change in time. 

And finally, the system energy – that is both kinetic and potential energy - must also be included in calculating the orbits: 

                                               (13).

Notice the value for E is only for masses 1 and 2; additional masses must have their own values, performed similar to equation 7. 

This is a lot of calculation! For one body in a system in one position, equations 8, 9, 10, 11, 12, and 13 must be performed. When the planet moves into a new position, a new series must be solved. This is why computer simulation is vital to simulating a system of planets. 

Computer simulation software comes in a variety of styles. Each code will utilize a specific style of mathematics to cope with the N-body problem. While description of these styles is beyond the scope of this paper, I will name a few as examples [R4]: 

• The Lagrangian Solutions
• The Jacobi Coordinate System
• Sundman and Levi-Civita Regularization
• Tisserand’s Criterion
• Bulirsch-Stoer Method
• Fourth Order T-U Symplectic Method
• Regularized Mixed variable Symplectic Method
• Wisdom-Holman Mapping

In many cases, a particular software model will utilize two or more of these methods as they can address specified portions of the N-body problem. 

Simulations for this project were performed using the SWIFT package written by Hal Levison and Martin Duncan. This particular package specializes in the use of massless test particles to study the effects by massive objects while not interfering with the massive objects themselves. SWIFT uses the following algorithms: 

• Bulirsch-Stoer Method
• Fourth Order T-U Symplectic Method
• Regularized Mixed Variable Symplectic Method
• Wisdom-Holman Mapping

The Bulirsch-Stoer method attempts to extrapolate the next step based on an initial step size[1]. The Fourth Order T-U Symplectic Method specializes in ecliptic orbits, but cannot vary step sizes. The Regularized Mixed Variable Symplectic Method specializes in close approaches to a massive body. And finally, the Wisdom-Holman Mapping encompasses the standard N-body parameters with a feature that corrects the orbit of Pluto.

SWIFT uses the Cartesian coordinate system for planetary setup. It is also limited to initial runs of 1,000,000 years, but can continue simulations beyond this point up to the limit. 

The image below shows an example of the initial planetary setup screen within SWIFT: 

Figure 2

Test particles (figure 3) are required in the SWIFT program, but the range is from 2 to 500 particles. The more particles, the longer the simulation runtime: 

Figure 3

Finally, the parameters screen provides selection for the runtime of the simulation: 

Figure 4

In the case of our simulations, test particles are not required but a good sample (10) will allow for the simulation to continue running in the event of planetary loss. In addition, the Integration Timestep is also a very important parameter as most exoplanetary systems have their orbits in days rather than years. A Timestep = 1 is an integration every year. In our case, a Timestep = 0.01 gave more accurate results but this limits integration time.

The Test Particle Conditions section is optional. 

Back to Top

4 – Solar System Simulations 

The works of Duncan & Quinn [R7] and Innanen et al. [R9] have demonstrated the long term stability of our Solar System out to a billion years. As such, the simulations of the Solar System will not confirm the obvious. Based on the works of Innanen et al. [R9], I will demonstrate the necessity for long term stability of the orbital eccentricities of the planets that include Earth and the inner Solar System. While the eccentricities do vary, the results of such a change did not grossly affect the overall stability of the Solar System as no bodies were ejected. It is accepted that the gas Giant planets provide the gravity to keep the system within the boundaries of the Solar System [R9].

The SWIFT simulator does contain the values for the 9 planets of the Solar System, but I found this lacking as the masses of the major moons and of the asteroid Ceres are not included in the sample. I changed the planetary setup to include 10 bodies, and moved the mass of the major moons to their host planet: 

While the simulations of the Gliese 876 system are sensitive to the initial setup parameters of the Cartesian coordinates, this was not the case in simulating the above Solar System. Once the masses were adjusted to compensate for the large moons, and with the introduction of Ceres, a stable simulation of the Solar System occurred 5 out of 5 times with random Cartesian coordinates out to 10⁷ years. 

The first set of simulations includes all 10 bodies – the color and number is to serve as a legend to the images: 

1. Mercury - Red
2. Venus - Green
3. Earth/Moon – Dark Blue (by serendipity)
4. Mars – Aqua
5. Ceres – Violet
6. Jupiter and the four Galilean Moons – Yellow
7. Saturn/Titan – Orange
8. Uranus – Green
9. Neptune/Triton – Blue-Green
10. Pluto/Charon – Blue

The simulation parameters were out to 1,000,000 years but the image below was constrained to a 100,000 year plot to allow the eccentric variability to be visible:

Figure 5

The values of the eccentricities of each of the planets above (figure 5) correspond well to the initial parameters. These results were duplicated five times. 

The second sets of simulations were run without the Earth/Moon mass. This simulation included 9 bodies: 

1. Mercury – Red
2. Venus – Green
3. Mars – Dark Blue
4. Ceres – Light Blue
5. Jupiter and the four Galilean Moons – Violet
6. Saturn/Titan – Yellow
7. Uranus – Orange
8. Neptune/Triton – Green
9. Pluto/Charon – Blue-Green

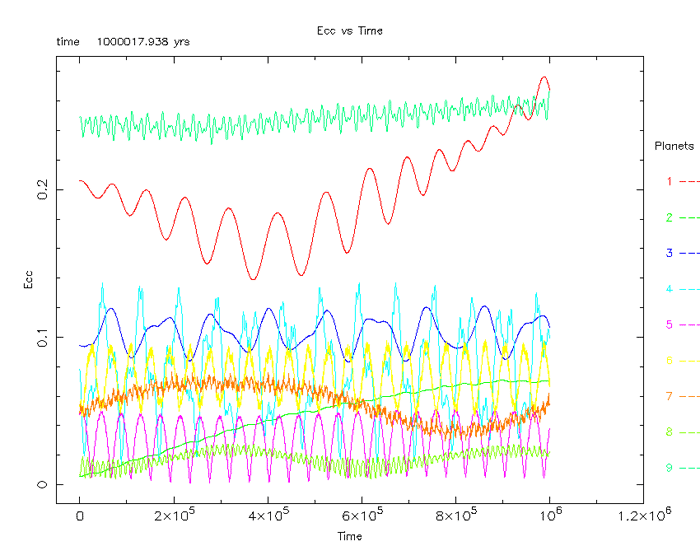
Figure 6

Again, this set of simulations (figure 6) was run five times with equal results. There are noticeable differences already between Pluto in the first simulation (Blue) and the second simulation (Blue-Green).  Also, the eccentricity of both Mercury (Red in both simulations) and Venus (Green in both simulations) begins to get larger in this simulation. Pluto also begins to migrate to a higher eccentricity. 

The final series of Solar System simulations only included Ceres and the outer planets: 

1. Ceres – Red
2. Jupiter and the four Galilean Moons – Green
3. Saturn/Titan – Blue
4. Uranus – Aqua
5. Neptune/Triton – Violet
6. Pluto/Charon – Yellow

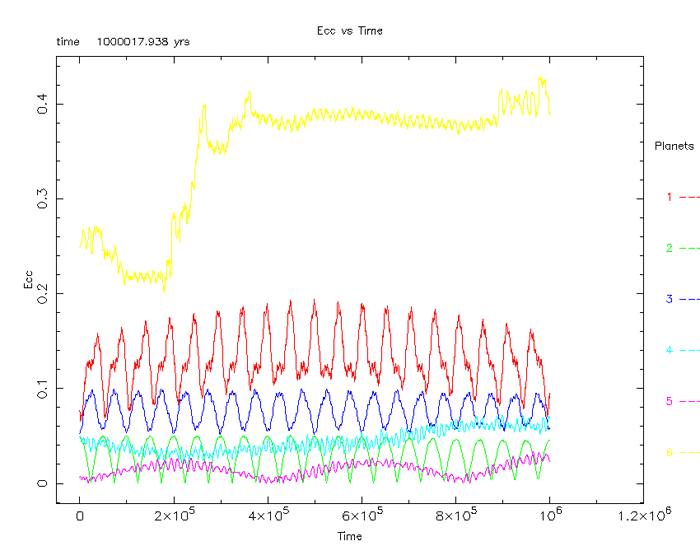
Figure 7

Immediately evident is the sharp increase in eccentricity of the orbit of Pluto (figure 7). Additionally, Ceres begins to assume a larger eccentricity as well. 

The parameters for the Solar System series: 

The test particles were random and set at 10. The test particle output was not plotted for this result.

Back to Top

5 – Gliese 876 Simulations 

The purpose of this paper is to determine the dynamical stability of GJ876d – the newly discovered planet – has on the 2/1 resonant stability of GJ876b and GJ876c. The gross computation of orbital resonance is the ratio of the orbital period of two bodies, in this case, GJ876b and GJ876c. With an orbital period of 61 days and 30.3 days respectively, both orbits can be reduced to a ratio of 2/1 – or at least close to it. The laws of orbits are applied here as the orbital duration is related to the distance the object is to the host star. Having said that, determining to stability of the 2/1 resonance of this system is the same as determining the overall orbital stability of the system. 

A painful lesson learned was that unlike our Solar System simulations, the initial setup of the Gliese 876 system is vitally important. Using Rivera et al. [R22] as a starting point, I was able to determine the initial Cartesian coordinate parameters. Given the time constraints of the project, I used an external source [R26] to convert the Keplerian coordinates – listed in the reference - to Cartesian.  

Keplerian coordinates [R22]:

Cartesian coordinates – converted [R26]: 

While the Keplerian coordinates provided by the literature resulted in stable results by the authors, the converted results for use with SWIFT was not successful. Two out of five runs failed to start while the other three revealed the bodies settling in to highly eccentric orbits[4]. I am suspecting the conversion process is not entirely accurate. In order to be epigrammatic, I have proceeded with my initial Monte Carlo simulations using random parameters until a stable system presented itself. 

The simulation parameters and test particle set-up remained constant for the duration of the Gliese 876 simulations: 

Test Particles:

Figure 8

Simulation Parameters:

Figure 9

The images below indicate a random selection of three simulations I performed on the Gliese 876 system. Each of the simulations has a different set of Cartesian coordinates, but the vital statistics are identical. 

The planet setup – Random 1:

Figure 10

The result of a 1000 year plot:

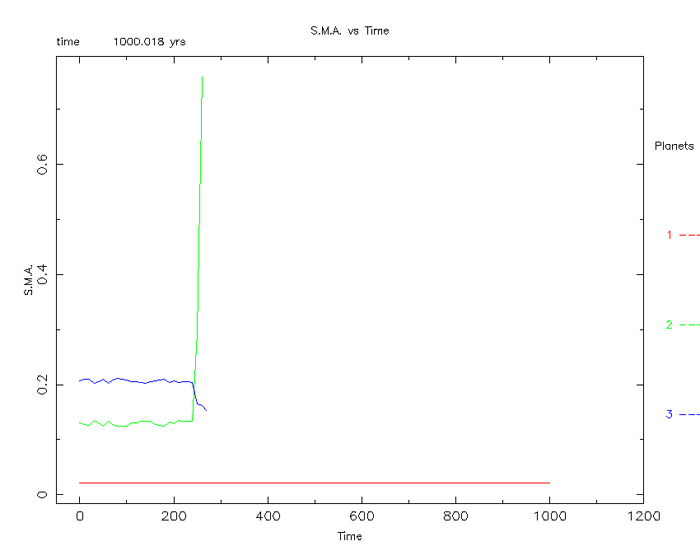
Figure 11

Almost immediately the two outer planets loose their “resonance” and take on very different orbits (figure 11). The middle planet has now become the systems outer planet as it quickly enters a large orbit. What is interesting however is the SWIFT simulator does not indicate the planet as “lost.” 

The Planet Setup – Random 2:

Figure 12

The result:

Figure 13

With only minor changes to the Cartesian coordinate set, the two outer bodies do maintain a stable resonance up to 900 years (figure 13). The end result is the same as the simulation above (figure 11). 

The Planet Setup – Random 3:

Figure 14

The result:

Figure 15

Our last random set and another change in Cartesian coordinates indicate a very unusual and highly chaotic system (figure 15). This type of orbit seems unlikely in reality. 

It is clear the initial location of the planets prior to running the simulation for this system is vital to overall stability. After numerous Monte Carlo simulations of a variety of Cartesian sets, a stable simulation was created: 

Figure 16

The result:

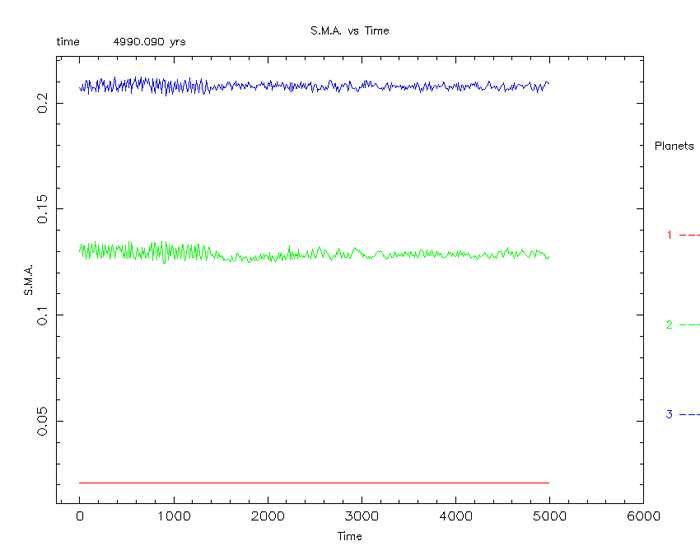
Figure 17

The image above (figure 17) demonstrates the stability of the Gliese 876 system to the full 5000 year simulation. There are obvious fluctuations indicated, but result from the natural resonant behavior of orbits in close proximity.

Now that we have our parameters, the goal now is to determine just how effective the inner planet is to the stability above. Keeping the parameters equal to figure 16, only changes in planet 1 are made. The first attempt is to reduce the mass of this planet. A simulation (figure 18) was run with planet one at a mass of 0.0001 M_J. 

Figure 18

The 5000 plot (figure 18) of this system reveals the inner body’s incapacity to remain in its orbits. With the reduced mass of planet 1, there is not enough counter-force keep the middle planet (planet 2) in position. 

Our second alteration of the mass or planet 1 is that of a small asteroid. The following simulation (figure 19) was run with planet 1 with a mass of only 0.000000001 M_J. 

Figure 19

The 5000 year plot of this system with the further reduced mass intensified the result of the inner planets incapacity to hold the middle planet. 

Various inclinations were explored in our Monte Carlo Cartesian sets, but this does not cover eccentricity. The circular orbit of the inner planet is also a stabilizing factor in this system. To demonstrate, the following simulation (figure 20) is of the planet setup values in figure 16 with only a change in eccentricity: 

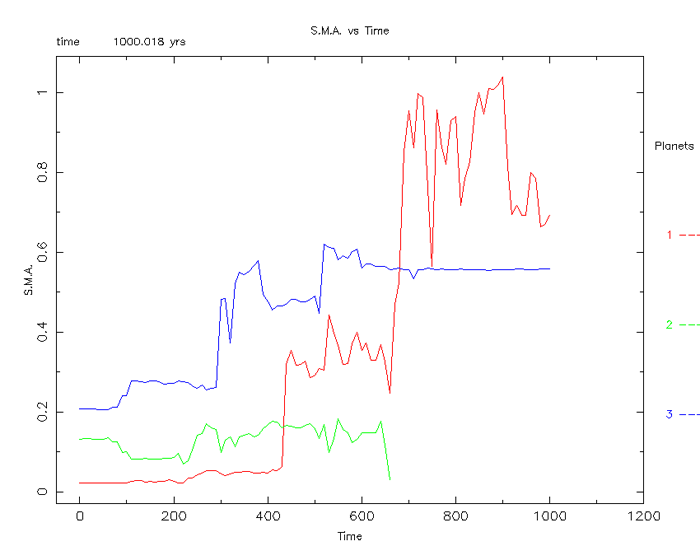
Figure 20

With an orbital eccentricity of planet 1 set to 0.1, it is found that the Gliese 876 system is not stable at all.

Figures 16 and 17 indicate our stable system. The following chart outlines the Cartesian coordinates and their counterpart Keplerian coordinates through conversion [R26]. 

Cartesian coordinates:

Keplerian coordinates:

Back to Top

Discussion 

The presence of the inner bodies in both the Solar System and the Gliese 876 system do affect the outcomes of the overall system dynamics. In the case of our Solar System, there are enough bodies spaced apart – and with significant mass – that our dynamic does remain in check but with changes to orbital eccentricity. In the case of the Gliese 876 system, the “perfect” 2/1 orbital resonance of GJ876b and GJ876c is vitally dependant on the inner planet – GJ876d. 

Resonance is an important component of the Gravitational Instability model [R24], the model used to describe the evolution of the exoplanetary systems. Our Solar System formed from the Solar Nebula Hypothesis. {During formation, these bodies that would form planets are called planetesimals. They begin their life as very small clumps of debris, but as the Solar Nebula contracts, a natural disk shape forms (a result of the conservation of energy - a natural phenomenon). These planetesimals assume their orbits and gather surrounding debris in a process called sweeping. Because the terrestrial planets form close to the proto-sun (the Sun at this point has not initiated fusion) the warmth melts away any ices so rocky planets form. The Gas Giants are at a greater distance and much of the ice and gas remains.}[5] 

The problem with the Solar Nebula Hypothesis is that is does not conform to any of the exoplanetary system that have been detected. The Gravitational Instability model is designed to compensate for this. According to this model, giant planet can form close to the host star but then settle into greater orbits equal to that of our own gas giant planets. In some cases, the movement of the giant planet can sweep up the necessary material to accumulate a gaseous atmosphere – that is if the system is young enough. This model also explains the resonant orbits of exoplanets as well: if two high mass planets are formed close to each other, they can lock together in resonance and maintain the same orbital dynamics for millions of years. 

In the case of Gliese 876, the large bodies exhibit a 2/1 resonance – that is the middle planet – GJ876c - orbits at 30.3 days while the outer planet - GJ876b - rotates at 61 days. It is believed the resonance of orbits can save smaller bodies – for example Neptune and Pluto’s 3:2 that has saved the smaller bodies such as TNO’s from ejection[6] – but our simulations shows this is not the case for Gliese 876d. It is possible that the 15/1 resonance of GJ876d and GJ876c provides the stability of GJ876c and GJ876b, but this requires more simulation. 

For the “perfect” 2/1 resonance to occur, the Gravitational Instability model was probably working as the large bodies began their migration. The inner planet could have provided the eccentricity required for GJ876c to allow GJ876b and GJ876c to be captured in a co-rotational resonance [R3][ R24]. Once resonance occurred, it should be noted that there will be certain libration to this resonance – in other words, each orbit may speed up or slow down as each mass acts on each other [R3] – similar to the action of a pendulum. 

Back to Top

Summary 

The lack of the Earth-Moon mass as well as the inner Solar System greatly affects the orbit of Pluto in our simulations. With the benefit of the gas giant planets of Jupiter, Saturn, Uranus and Neptune, the residual bodies of the Solar System are still in check. The planetary system of Gliese 876 does not have the luxury of multiple large bodies to keep the system stable. In fact, the orbital resonance of the two large bodies at such close proximity relies heavily on the presence of the inner planet. There is enough mass within the inner body to provide the counter-resonance required to keep this system in check. It is difficult to say if the 15/1 ratio of the inner two bodies is required for the 2/1 ratio of the outer two bodies - only with further computer simulation can we determine this to be true – but the simulation results seems to support this idea. 

I would like to thank my supervisor, Professor James Murray, for putting up with my endless simulation questions and for helping me through the simulations of the Gliese system, and for help in understanding the mechanics involved between the Keplerian and Cartesian system of coordinates. I would also like to thank the Swinburne Astronomy Online group for providing the material so this class could be taken and I would also like to thank the folks over at the Swinburne Supercomputer section for allowing the students to run the simulations required to complete this class.

Back to Top

References 

[R1] Aarseth, Sverre. Gravitational N-Body Simulations. Cambridge Monographs on Mathematical Physics, Cambridge University Press. 2003. 

[R2] Beauge, C.; Ferraz-Mello, S. and T.A. Michtchenko. “Planetary Migration and Extrasolar Planets in the 2/1 Mean-Motion Resonance.” ADS Pre-Print, April 2004. 

[R3] Binney, James and Scott Tremaine. Galactic Dynamics. Princeton University Press, New Jersey. 1987. 

[R4] Boccaletti, D. and G. Pucacco. Theory of Orbits. 1: Integrable Systems and Non-perturbative Methods. Springer, Berlin. 2001. 

[R5] Carpino, M.; Milani, A. and A.M. Nobili. “Long-Term Numerical Integrations and Synthetic Theories for the Motion of the Outer Planets.” Astronomy and Astrophysics, 181:182-194. 1987. 

[R6] Carrol, Bradley and Dale Ostlie. ­An Introduction to Modern Astrophysics. Addison-Wesley Publishing Company, Massachusetts. 1996. 

[R7] Duncan, Martin and Thomas Quinn. “The Long-Term Dynamical Evolution of the Solar System.” Annual Review of Astronomy and Astrophysics, 31:265-295. 1993 

[R8] Hoi, Man and S.J. Peale. “Extrasolar Planets and Mean-Motion Resonance.” Astronomical Society of the Pacific Pre-Print, September 2002. 

[R9] Innanen, Kimmo; Mikkola, Seppo and Paul Wiegert. “The Earth-Moon System and the Dynamical Stability of the Inner Solar System.” The Astrophysical Journal, 116:2055-2057. October 1998. 

[R10] Ji, Jianghui; Li, Guangyu and Lin Liu. “The Dynamical Simulations of the Planets Orbiting GJ 876.” The Astrophysical Journal, 572:1041-1047. June 2002. 

[R11] Ji, Jianghui et al. “The Liberating Companions in HD 37124, HD 12661, HD 82943, 47 Ursa Majoris, and GJ 876: Alignment or Antialignment?” The Astrophysical Journal, 591:L57-L60. July 2003. 

[R12] Jones, Barrie W. and Nick Sleep. “The Orbits of Terrestrial Planets in the Habitable Zones of Known Extrasolar Planetary Systems.” Astronomical Society of the Pacific Pre-Print, November 2002. 

[R13] Kinoshita, Hiroshi and Hiroshi Nakai. “Stability of the GJ 876 Planetary System.” Astronomical Society of Japan, 53:L25-L26. August 2001. 

[R14] Kley, W. “Dynamical Evolution of Planets in Disks.” Kluwer Academic Publishers. Netherlands, 2003. 

[R15] Laughlin, Gregory et al. “The GJ 876 Planetary System: A Progress Report.” The Astrophysical Journal, 622:1182-1190. April 2005. 

[R16] Marcy, Geoffrey et al. “A Planetary Companion to a Nearby M4 Dwarf, Gliese 876.” The Astrophysical Journal, 505:L147-L149. October 1998. 

[R17] Marcy, Geoffrey et al. “A Pair of Resonant Planets Orbiting GJ 876.” The Astrophysical Journal, 556:296-301. July 2001. 

[R18] Mayor, Michael and Pierre-Yves Frei. New Worlds in the Cosmos. The Discovery of Exoplanets. Cambridge University Press, 2003. 

[R19] Pasachoff, Jay and Alex Filippenko. The Cosmos: Astronomy in the New Millennium – Second Edition. Thompson Brooks/Cole, Australia. 2004. (Note: material from this reference is from the online bonus material, available to purchasers of the book only – and for a limited time). 

[R20] Psychoyos, Dionyssia and John D. Hadjidemetriou. “Dynamics of 2/1 Resonant Extrasolar Systems Application to HD82943 and Gliese876.” Kluwer Academic Publishers. Netherlands, 2004. 

[R21] Rivera, Eugenio J. and Jack J. Lissauer. “Dynamical Models of the Resonant Pair of Planets Orbiting the Star GJ 876.” The Astrophysical Journal, 558:392-402. September 2001. 

[R22] Rivera, Eugenio J. et al. “A `7.5 Earth-Mass Planet Orbiting the Nearby Star, GJ 876.” ADS Pre-Print, 2005. 

[R23] Snellgrove. M.D.; Papaloizou, J.C.B. and R.P. Nelson. “On Disk Driven Inward Migration of Resonantly Coupled Planets with Application to the System Around GJ876.” Astronomy and Astrophysics, pre-print. April 2001. 

[R24] Zhou, J.L.; Aarseth, S.J.; Lin, D.N.C. and M. Nagasawa. “Origin and Ubiquity of Short-Period Earth-like Planets: Evidence for the Sequential-Accretion Theory of Planet Formation.” The Astrophysical Journal Letters, August 2005. 

Back to Top

Image Credits 

Figure 1: http://scienceworld.wolfram.com/physics/OrbitalElements.html 

Internet Resources

Astronomy Online: http://astronomyonline.org/Exoplanets/Exoplanets.asp 

[R25] SWIFT Website: http://www.boulder.swri.edu/~hal/swift.html 

[R26] Changing of the Elements: http://janus.astro.umd.edu/orbits/elements/convertframe.html  

Back to Top