|
Modeling Core Collapse Supernova - by Alex Nervosa:
Back to
High Mass Stellar Evolution
Introduction
Overview of SNe Theoretical Models
One Dimensional Models
2-D Models
3-D Models
The Role of Stellar Rotation in Multidimensional Modelling & Observations
Nucleosynthetic
Chemical Yields & Mass Ejection
Physics of Core Collapse
Supernova
Nucleosynthesis of Type 1a SNe
Type Ib, c, II SNe Nucleosynthesis
Neutron Capture,
Radioactive Decay and Observations
Particle & Neutrino Physics
Conclusion
References
Credits and Comments
Back to
High Mass Stellar Evolution
Introduction
Theoretical stellar models that attempt to reproduce supernovae (SNe1) via core collapse processes are embodied in numerical simulations. Recent advances in our understanding of SNe processes as well as computer technologies both hardware and software, are producing increasingly sophisticated multi-dimensional SNe simulations attempting to reproduce observational results. Our discussion will contrast the different types of simulations used, associated input physics, reproduced chemical yields and ejected mass as well as key nucleosynthetic, particle physics and neutrino formation processes. In our discussion we’ll also attempt to correlate theoretical results with recent SNe observations and discuss the implications and futures of SNe theoretical models.
Back to Top
|
Back to
High Mass Stellar Evolution
Overview of SNe Theoretical Models
Modelling core collapse SNe is an active field of research that is rapidly maturing. It has been shown that Hydrodynamical bounce-shock energy transport following core collapse is on its own not sufficient to drive SNe explosions [7, 14]. Multidimensional simulations (2-d and 3-d simulations) representing theoretical SNe models have improved to the point where they are able to reproduce delayed explosions via neutrino driven energy transport and deposition effects. Multidimensional simulations still require approximations in input physics as technology and our understanding of the physical processes involved in SNe are evolving.
SNe stellar models attempt to simulate dynamic changes in stellar structure around core collapse time so that chemical yields, mass ejected and related explosive nucleosynthesis processes may be analysed. A simplified SNe structural model is shown in Figure 1:

The stellar core is composed of a forming proto-neutron star, with an outwardly expanding neutrinosphere. The core is surrounded by an expanding mantle with an outer edge that forms a shockfront boundary between expanding matter and accreting mass from adjacent stellar layers. Convection is thought to form in the mantle region and within the neutrinosphere.
Back to Top
|
Back to
High Mass Stellar Evolution
One Dimensional Models
One dimensional (1-d) models are spherically symmetrical,
non-rotational representations of progenitor stars2
which were originally
pioneered in simulations by Colgate & White in 1966 [30] and Arnett in 1967
[31]. The basic foundations on which subsequent 1-d models evolved (as well as
2-d and 3-d models) were effectively provided by these authors. The Colgate,
White & Arnett’s 1-d models used many approximations of neutrino-driven energy
transport, neutrino physics as well as equations of state so as to demonstrate
how SNe may be created.
Throughout the 1960’s and early 1970’s there were fundamental
problems with 1-d models that precluded a physically complete description of
core collapse SNe. There was a lack of realistic stellar progenitor models.
There was also a neglect of weak neutral currents as well as uncertainties in
the equation of state at super-nuclear densities [48]. Aside from input physics
limitations from a technology perspective, there wasn’t any real ability to
experiment beyond the spherically symmetrical, static (non-rotational) 1-d
models.
Some of the characteristics of 1-d models include the emphasis
on the neutrinodriven process as the means to drive the SNe explosion such that
neutrinos emanating from the neutrinosphere deposit gravitational energy from
the stellar core in the SNe mantle (see figure 1). Improvements in input physics
relating to neutrino energy transport for instance using various Boltzmann
solvers [7], has improved and provided a more accurate representation of the
neutrino energy transport mechanism, however this hasn’t altered the overall
imploding characteristics of 1-d models.

These characteristics are such that most 1-d models don’t
demonstrate a net explosion and appear to fizzle into quasistatic accreting
proto black holes [17] as shown in figure 2. Additionally, there doesn’t seem to
be a widely accepted method that may contribute to the revival of the stalled
shock observed after initial explosion. Even with up-to-date nuclear equations
of state, neutrino physics such as accounting for the neutrino oscillation
effect, improved stellar progenitor models, various implementation methods of
1-d simulations and up-to-date energy transport algorithms [23, 24, 25, 26] when
using either a low or high entropy stellar core model, the energy coupling
efficiency between the SNe core and mantle (core and mantle shown in figure 1)
governed by neutrino-matter interactions is inadequate in 1-d models to prevent
stalling of core-bounce shock explosion and its subsequent reignition. This
leads to a recollapse even after a pause or ‘delayed’ re-ignition in 1-d models.
It’s evident also that this failure is consistent with various
stellar progenitor models [17] such that varying the source model parameters
doesn’t greatly affect the implosion outcome. The implications are clear. No
explosions are generally observed in 1-d for Type I or Type II stellar
progenitor models.
There has been evidence presented indicating that certain 1-d
models do indeed show an overall net explosion after a delayed phase [32, 33,
34, 35]. These results are however controversial as neutron-finger convective
instabilities and nuclear equations of state with high abundances of pions [7],
used as methods to boost neutrino luminosities have been widely questioned by
the astronomical [18] and nuclear physics [7] community, as they are seen to be
unlikely contributors to the required energy deposition process required to
drive and maintain SNe explosions.
Back to Top
|
Back to
High Mass Stellar Evolution
2-D Models:
Technological advances in the 1980’s coincided with pioneering
SNe observations such as SN 1987A, which indicated anisotropies3
and large scale mixing of elements [14]. Armed with these constraining
observations, 2-d models began to be used by some research groups aiming to
overcome the stalled shock phenomenon observed in 1-d models [18]. One of the
main differences of 2-d models when compared with 1-d models is that they take
into account stellar rotation which brings on convection in the mantle region
(figure 1). Figure 3 shows how rotational effects, more specifically the
introduction of angular momentum, affects the entropy of theoretical SNe (shown
in red).

Although 2-d models use similar input physics as 1-d models such
as nuclear equations of state and neutrino nuclear cross-sections in
calculations, compromises are generally made. For instance full neutrino
transport is quite difficult to model in multi dimensional algorithms [7, 17],
hence simple approximations in 2-d models are usually incorporated such as grey
(spectrally averaged) energy transport and flux-limited, energy diffusion
transport mechanisms [1, 3, 7].
Notwithstanding these approximations, initial results of 2-d
models are promising, showing overall net explosions (as opposed to 1-d models)
as indicated in figure 3. However 2-d (and 3-d models) are still not able to
reproduce all the important observational signatures required to constrain and
validate these models [17], which currently remains an outstanding problem in
stellar astrophysics. We will explore some of these observational signatures
later in our discussion.
A key difference brought about by rotationally induced
convection of 2-d models is the efficiency of neutrino-matter coupling and the
neutrino energy deposition rates observed in the results. Both of these are
fundamentally related to stellar convection occurring in the ‘gain region’
(which is a thermal boundary in the mantle inside the shock front) [27, 41]
where neutrino processes take place. Essentially in 2-d models, mantle
convection makes the protoneutron star unstable. The resulting increase in
entropy leads to a higher overall unstable core and mantle regions. All of this
happens whilst processes outside the shockfront such as accretion and accretion
induced luminosity continue to act against the shockfront. The net result after
milliseconds from core bounce is a delayed SNe explosion [17]. Calculations
performed in 2-d models point to a more unstable mantle due to convection;
however this also appears to lead to weaker explosions when contrasted with
observations [17, 28, 29]. It’s becoming clear however that the presence of
convection, coupled with neutrino induced heating mechanisms ensures explosion
of 2-d models [29].
Convection-induced perturbations in particle velocity and
neutron excess (Y_e) can also alter the nature of 2-d core collapse models
compared to the spherically symmetric 1-d models [3] favouring a SNe explosion.
Explosive nucleosynthesis via neutrino induced heating in the oxygen layer at
the bottom of the convection region where the forming proto-neutron star is
located, also appears to be responsible for the success of 2-d explosion models
[1]. Iron peak isotopes are created in this region such as Ni-56, responsible
for powering SNe light curves. Explosive nucleosynthesis at the bottom of the
oxygen convective shell is also responsible for the production of gamma ray
emitting radionuclides such as Ti-44, Ni-57 and Co-56. Perturbations in neutron
excess density and temperature that are left over from convective oxygen shell
burning greatly affect the relative abundances of created isotopes. For example
even the slightest change in neutron excess to the order of 10^(-4) can change
the relative abundance of Fe-57 to Fe-56 by a factor of about 2 [3].
Recent advances in technology coupled with an improved
understanding of the physics involved may allow neutrino transport to be handled
in hardware and software using multi-group, multi-angle (Boltzmann solvers)
techniques and also that hydrodynamical transport be handled in a similar way by
means of solving general relativity equations [17]. Essentially this is about
bringing more detailed microphysics into 2-d (and 3-d) models to avoid using
approximations such as grey energy transport and flux-limited energy diffusion
techniques. Preliminary results however don’t appear to significantly change
from those of ‘conventional’ 2-d (and 3-d) models (those without the 1-d
microphysics) which make use of energy transport approximations [2]. The
implications of this may be that input microphysics may not add much more to the
high level view of how SNe explosions take place and evolve, as opposed to
rotation related effects.
Back to Top
|
Back to
High Mass Stellar Evolution
3-D Models:
3-d stellar models allow the representation of input physics and
dynamic range required in a more comprehensive and holistic manner that may be
performed in 2-d simulations. Based on 1-d and 2-d experiences, consensus in the
astronomical community is that rotation and related effects induced, such as
convection play a key role in SNe explosions [7]. It follows then that the need
for 3-d simulations has been driven by the desire to more accurately model
stellar objects in real life [6, 10], hence the requirement to use all
dimensions available. This implies modelling a spherical body without boundary
conditions [6] which is an inherent limitation of 2-d stellar models.
Besides the dynamic range and more accurate convection modelling
available in 3-d, there are other relevant real-to-life processes that may be
accounted for and more accurately modelled in 3-d, such as: explosion
asymmetries which arise due to differences in convective regions [9]. Other SNe
features that benefit from a 3-d treatment are convection asymmetries in the
neutrinosphere [36], neutron star kicks [1, 21], magnetic fields, core
fragmentation, convective instabilities, gamma-ray spectra produced and
nucleosynthetic yields [10, 36]. In addition, angular momentum is also able to
be more accurately modelled as it has been shown to pose constraints on
convection efficiency, particularly in the equatorial region of progenitor
models. It is important to note however that to achieve all this in 3-d within a
reasonable timeframe requires hardware to be computationally expedient and
software algorithms to achieve a balance between speed vs. additional
sophistication required to consider these SNe effects.

Over the last 10 years 3-d simulation have provided results with
interesting and at times controversial conclusions. For instance 3-d models of
subsonic nuclear burning (deflagration models) in Type Ia SNe that have no
adjustable parameters (such as initial flame geometry and multiple ignition
spots outside the core as shown in figure 4) may produce weaker explosion energy
models than 2-d Type Ia SNe counterparts. However nucleosynthetic predictions
such as the produced masses of Nickel and ejecta velocities fall within
acceptable observed ranges, unlike their corresponding 2-d models [5]. The
implications of this for 3-d models and SNe Type Ia is that no fine tuning of
the initial model is required in order to reproduce relevant observations as
they relate to masses and ejecta.
When this is contrasted with Type Ib, c and Type II SNe a
similar result is noticed in relation to explosion energy i.e. weaker explosions
in 3-d models when compared to 2-d models [6] which is inconsistent with
observations. Results also indicate that 3-d models may indeed produce higher
explosion energies for Type Ia SNe [13] as opposed to Type Ib, c and Type II
SNe. The dynamic nature of 3-d simulations introduce numerical uncertainties
such as artificial density variations in the progenitor stellar model and
numerical shear introduced by artificial viscosity typical in 3-d numerical
algorithms [10]. Although further work is required in order to refine 2-d and
3-d models such as explaining the overall nature of delayed and weaker
explosions, the outlook compared to 1-d models appears to be promising in
relation to observations.
Back to Top
|
Back to
High Mass Stellar Evolution
The Role of Stellar Rotation in Multidimensional Modelling &
Observations
Aspects related to theoretical rotating stellar models are
explosion asymmetries, increased and reduced convection in polar and equatorial
regions respectively and assistance in providing and sustaining neutrino driven
energy transport and energy deposition. These aspects are directly related to
SNe observations.
Stellar rotation as a catalyst for convection and neutrino
induced heating assists the expansion of SNe shock front in polar regions.
Rotationally induced centrifugal forces resist gravitational collapse at the
equator from mass accretion whilst increasing the efficiency of convection at
the poles, hence easing the progression of the neutrino powered shock front in
polar regions [7]. Although asymmetric SNe explosions may be of a delayed nature
and lesser energy in rotating models (2-d and 3-d) the increased convection
efficiency at the poles through rotation is able to greatly increase neutrino
heating efficiency [1]. This results in explosion asymmetries.
Explosion asymmetry will cause deeper mixing in SNe ejecta and
it has been shown also that polarization, as an observational signature [37] is
consistent with asymmetric explosions [1, 37] in both Type I and Type II SNe.
Hence asymmetric explosions of SNe may contribute to the extended mixing of
elements and anisotropy observed. For instance observed extended mixing of iron
peak elements may be explained by matter ejected along the poles with a much
higher velocity as is the case in multidimensional rotational stellar models
[37].
Rotational effects also appear to impact on overall neutrino
luminosities. For instance non-rotating stellar models have larger neutrino
luminosities as opposed to their rotating counter parts. This is due to
non-rotating stellar core demonstrating an increase in gravitational compression
as well as a large exponential neutrino energy effect due to temperature
increase. For instance pair annihilation, which is a process that produces
neutrinos begins at temperatures of 10^9 Kelvin [1]. The time of explosion is
also earlier for a non-rotating stellar cores by a minimum factor of 2 [1].
Despite the differing results around explosion energies which
require more experiments to fully resolve, it’s becoming clear that rotational
models when compared to non-rotational models, induce asymmetries in SN
explosions via convection, resulting in ejecta travelling faster at the poles
than at the equator. This effect can be quite significant and is confirmed with
given observations – the ratio of polar ejecta velocities to equatorial
velocities may be in the order of 2 [1], consistent with theoretical results.
Other aspects of neutrino processes consistent with convection resulting from
rotation are an increase in luminosity from beneath the neutrinosphere,
increased neutrino absorption close to the shockfront and energy transport and
deposition occurring well away from the neutrinosphere [48]. These convection
driven aspects assist in producing asymmetries and convection instabilities in
theoretical multidimensional models.
Back to Top
|
Back to
High Mass Stellar Evolution
Nucleosynthetic Chemical Yields & Mass Ejection
Multidimensional simulations are able to assist in predicting
chemical yields and mass ejected in the interstellar medium (ISM), however
simulations need to consider two ways in which nucleosynthesis takes place in
the context of stellar evolution and SNe. The first is conventional
nucleosynthesis via fusion reactions occurring via the creation of heavier
elements up to Iron resulting from increasing core temperatures during a SNe
progenitor’s life. The second method is explosive nucleosynthesis which takes
place when a hydrodynamical (Type Ib, c, and Type II SNe) or thermonuclear (Type
Ia SNe) explosion occurs within the progenitor’s core. The most important one is
explosive nucleosynthesis in the context of core collapse SNe.
Once a hydrodynamical or thermonuclear explosion takes place, a
shock wave travels quickly from the core area outward into the adjacent inner
stellar layers. Element creation via nucleosynthesis reactions takes place
instantaneously as the travelling shock forces its way from the central core.
Numerical simulations indicate that initially only elements residing in the core
and adjacent inner layers are affected by explosive nucleosynthesis whilst
leaving elements residing in the outer stellar layers relatively untouched by
this process until the shock wave finally reaches the outer layers [38].
Theoretical stellar models that produce chemical yields and mass
ejected as byproducts of SNe are dependent on contributing aspects such as input
physics and explosive nucleosynthesis used. Each aspect has a range of
parameters that astrophysicists need to carefully consider that will ultimately
have a degree of impact on chemical yield produced and mass ejected. The
following table shows an example of some of the parameters that require
consideration for stellar models attempting to reproduce chemical yields and
associated mass ejected:

As a result of the varying combinations that may be used in
calculating chemical and mass ejection yields, it is common for results to vary,
and in some cases even significantly [38]. As an example multidimensional
simulations (2-d and 3-d) may provide yield differences compared with 1-d
simulations which although negligible for light elements, are somewhat
noticeably different for heavier elements [11, 12]. This is generally attributed
to the consistently higher temperatures produced by multidimensional simulations
in the inner core induced by stellar rotation (hence convection related
effects), which leads to higher core neutronization (neutron and neutrino
production). Core neutronization assists in the production of isotopes that are
very sensitive to neutron capture such as those of Calcium, Titanium, Vanadium,
Chromium and Zinc [11].
It is important to note however that amongst the differences in
chemical yields and hence mass ejected between models and various approaches to
input physics, there are commonalities. For instance in hydrodynamic explosions
characterised by Type Ib, c & Type II SNe one group of elements, which include
Oxygen, Neon and Magnesium are not generally affected by explosive
nucleosynthesis. These elements are created in the outer layers well away from
the ignition point during conventional nucleosynthetic processes. This group of
elements have yields proportional to the mass of the progenitor star and not
dependant on explosive nucleosynthesis. It follows then that massive
hydrodynamically ignited SNe eject more elements from the outer layers than less
massive SNe of the same type. Conversely another group of elements that include
Iron, Calcium, Sulphur and Argon are produced as a direct consequence of
explosive nucleosynthesis and to a degree are less dependent on progenitor mass
[38, 11].
Figure 5 summarises work performed by various research groups in
providing Oxygen and Iron estimates for theoretical Type II SNe, which we’ll use
as indicative examples of elements in the two groups described earlier. One can
clearly see that for Oxygen the mass ejected on the y-axis in solar masses, as a
function of progenitor mass (x-axis) clearly increases across all models used.
This is in contrast to Iron mass ejected for each computed model, indicating
that for Type II SNe, Iron doesn’t readily pollute the ISM and is retained as
part of the post-explosion stellar corpse.
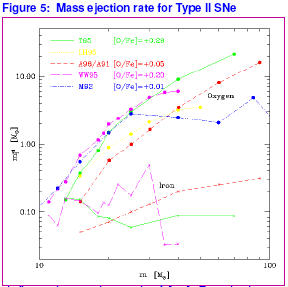
These theoretical results from modelling can be contrasted with
observations such that they may be validated in order to constrain or otherwise
the contributing aspects of multidimensional input physics and explosive
nucleosynthesis.
Recent multidimensional modelling of Type Ia SNe such as those
shown in figure 4, have made use of nonadjustable inputs for simulated
thermonuclear explosion processes(as opposed to 1-d models) in order to examine
chemical yields and mass ejection quantities as a function of explosion type
such as deflagration or detonation4 [12]. Results have shown
that large amounts of Iron are produced and that the core Carbon-to-Oxygen (C/O)
ratio in Type Ia SNe progenitors affects the amount of Ni-56 produced (the more
unburnt Oxygen remains, the less Ni-56 created) which has implications not only
for chemical yields and related mass ejected, but also for Type Ia SNe energy
generated and detected via light curve analysis [39]. Consequently it’s
reasonable to assume that chemical yields produced, energy generated and mass
ejected are a function of progenitor composition, temperature and
nucleosynthesis for Type Ia SNe [12].
Theoretical multidimensional models and observations for ionised
Iron in Type Ia SNe are generally consistent [4]. Multidimensional models of
Type Ia SNe have shown also that much larger fractions of unburnt Carbon and
Oxygen are retained after a thermonuclear explosion by the ISM (up to as high as
50%) which is 2.5 times more than 1-d simulations [12]. Light curve observations
provide the ability to constrain or otherwise these results produced [22, 39]
which appear to be high in multidimensional models compared to observations [4,
20].
Advances in multidimensional models are able to provide an
insight into certain elements that are very sensitive to explosive
nucleosynthesis such as Ni-56 and Ti-44. Both these elements are extremely
important for the evolution of SNe remnants and for the subsequent chemical
evolution of galaxies [11]. Elements such as Ni-56 and Ti-44 are also useful as
‘simulation probes’ as their yields and mass ejection rates can assist in
constraining numerical models, more specifically hydrodynamical effects and
neutrino-matter interactions, which ultimately assist in better understanding
thermonuclear (Type Ia SNe) and hydrodynamical (Type II SNe) explosions.
Although substantial work has been done with 1-d and
multidimensional simulations in an attempt to reproduce observations, more work
is required to further refine these models. An example of this is the neutron
excess (Y_e) problem. Y_e is constrained by nucleosynthesis and is generally
higher than allowed for 1-d models and higher again for multidimensional models
infact, Type Ib, c and Type II SNe 2-d and 3-d models leave behind neutron stars
which are lower in mass than observations put forward [48]. This suggests that
other input physics and/or explosive nucleosynthesis considerations need to be
accounted for to take this observational aspect into account [1].
Although there are discrepancies in 1-d and multidimensional
results and observations as they relate to yields and mass ejected, improved 3-d
modelling techniques are thought to be able to reduce the discrepancy between
theory and observations such that more efficient burning of the theoretical C/O
core may take place [19] as an example. Notwithstanding discrepancies, the
general consensus in the astronomical community appears that 3-d simulations
(given their ability to better represent dynamic range and “real life” effects)
are able to more faithfully reproduce chemical yields, mass yields and observed
light curve spectral features than 1-d or 2-d models [4, 19], hence this sets
the scene for the future of 3-d simulations as the bridge between theory and
observations.
Back to Top
|
Back to
High Mass Stellar Evolution
Physics of Core
Collapse Supernovae
It is appropriate at this point to discuss relevant
nucleosynthesis, particle physics and neutrino 5
processes with particular
focus around core collapse events. Type Ia and Type II (including Type Ib, c)
SNe explode via thermonuclear and gravitationally induced core collapse
respectively, and each also undergo conventional and explosive nucleosynthesis6 before and after core
collapse. These will be covered for each SNe type in conjunction with relevant
particle physics and neutrino processes. Differences will be highlighted where
appropriate in the following discussion.
Nucleosynthesis of Type
Ia SNe
Element nucleosynthesis via fusion processes in Type Ia SNe
(progenitor solar mass < 8 solar masses [38]) is able to produce elements up to
Carbon as shown in figure 6. Key fusion reactions responsible for the stellar
structure in figure 6 are the Proton-Proton chain and the Carbon-Nitrogen-Oxygen
chain, simply described in table 2 as follows:

Both the PP and CNO reactions occur at increasingly higher core
and inner layer temperatures. These reactions are able to produce heavier
elements in respective layers closer and closer to the core as shown in figure
6. At a point at which electron degeneracy doesn’t allow pressure to increase
further, the C/O core temperature continues to increase until it explosively
ignites via detonation and/or deflagration [12]. At this point explosive
nucleosynthesis along with neutron capture [42] is responsible for the
production of radioactive isotopes of elements such as Silicon, Nickel, Titanium
and Iron, which are subsequently ejected in the ISM [12].

Although neutrino processes occur during conventional and
explosive nucleosynthesis, neutrino energy deposition plays a small part in
powering the explosion of Type Ia SNe, given that neutrinos easily disperse away
from the stellar core [49]. Radioactive decay of elements via gamma ray and beta
decay occur [44] which contribute to the observed energy deposition rates,
chemical and mass yields and element abundances detected in Type Ia SNe light
curves.
Back to Top
|
Back to
High Mass Stellar Evolution
Type Ib,c,II SNe
Nucleosynthesis
When the Type Ia SNe scenario is contrasted with more massive
progenitor stars (Type Ib, c & Type II SNe) we find a different structure and
more evolved conventional nucleosynthesis before core collapse. Element
nucleosynthesis via fusion in progenitor stars with solar mass > 10 solar masses
[38] are able to produce elements up to Iron as shown in figure 7 below. The key
fusion reactions (in their simplified form) which occur with increasing core and
inner layer temperatures are shown in table 3 as follows:


Once the Iron core is formed, neutrino processes in Type Ib, c &
Type II SNe (unlike Type Ia SNe) become very important. As neutrinos are
transparent to the stellar core they radiate away, leading to
photodisintegration of the core which results in further gravitational core
collapse. Ultimately this leads to core rebound at nuclear density generating a
shock wave powered by a second wave of neutrinos which meets gravitationally
infalling matter. At this point explosive nucleosynthesis takes place, freezing
out the newly created elements via numerous nuclear reactions. Elements are
created such as Nickel, Titanium, Cobalt and Sodium. These and other radioactive
isotopes created via neutron capture processes, decay with varying ˝ lives.
Subsequently energy released in element decay, powers observed SNe light curves
which are used to spectroscopically determine element abundances, energy
deposition rates in circumstellar material, chemical yields and mass ejection
rates.
Back to Top
|
Back to
High Mass Stellar Evolution
Neutron capture, radioactive decay and observations
During a SNe explosion neutron capture takes place which can
occur via the slow process (s-process) or via the rapid process (r-process)
[40]. The neutron capture rate (slow or rapid) is determined relative to
competing beta decay rates. Hence in the s-process neutrons are captured slowly
by elements relative to the beta decay rates and in the r-process neutrons are
captured faster by elements relative to beta decay rates. Although both these
processes occur in SNe, the r-process dominates over the s-process meaning that
neutron capture is extremely efficient. This is demonstrated in both 1-d
simulations [42] and multidimensional simulations [6,16], and is also thought to
be responsible for the majority of existing elements beyond Iron as shown in the
periodic table [43]. The r-process is also responsible for producing some of the
heaviest elements such as the transuranic elements beyond Bi-209 [40], which is
not possible by the s-process. The following table shows the generalised nuclear
reactions for each competing processes namely neutron capture and beta decay.

A key radioactive decay process which follows explosive
nucleosynthesis in both Type I and II SNe is Ni-56 -> Co-56 -> Fe-56, which is
responsible for gamma ray and beta decay energy deposition in SNe light curves.
This reaction is directly responsible for sustaining the energy observed in SNe
light curves.

It’s important that if theory is to be validated by
observations, that simulations (particularly multidimensional simulations as
these have so far demonstrated to be able to better predict observations [15])
improve to the point of homologous expansion [8] so that synthetic spectra
produced via initial conditions can be reasonably verified with observational
SNe light curves.
To this end various research groups [5, 12, 13, 15] are
undertaking this task. Figure 8 shows synthetic light curves (solid black) vs.
observed light curves (dotted green) for Type Ia SNe. In general the synthetic
approach is on the right track, however more work is still needed to explain
discrepancies and required to fine tune synthetic spectra.
Back to Top
|
Back to
High Mass Stellar Evolution
Particle & neutrino
physics
Several particle7 and nucleon8
processes in SNe physics
contribute to neutrino production, absorption and neutrino scattering. These
processes are particularly important for heavier stellar progenitors of Type Ib,
c, and Type II SNe around core collapse time as neutrino interactions are
essential in producing and powering SNe explosions.
An important producer of neutrinos is neutronization9 which is a nucleon
process based on electron capture. At the point of nuclear degeneracy and core
bounce in SNe, neutronization recombines protons and electrons to create
neutrons and electron-neutrinos (free protons and electrons in the core exist as
a result of photodisintegration). Neutronization occurs alongside
electron-antineutrino production which is another electron capture process,
whereby electrons combine with neutrons to create electron-antineutrinos and
protons inside the gain radius. These two processes cool the outside of the
forming proto-neutron star as neutrinos diffuse away from the core. This is
shown in table 5 and figure 9 where it’s described as the ‘cooling’ region.

Outside the gain radius neutrinos encounter high opacity at the
shockfront due to expanding and infalling matter meeting at this point10.
This zone, called the heating region (see figure 9) is where neutrino absorption
takes place (as shown also in table 5).
SNe energy gained by neutrino absorption in the heating region
is greater than energy lost by electron capture in the cooling region [41].
Hence energy deposition via neutrino absorption in the heating region is a key
factor in driving and reviving stalled SNe shocks that attempt to propagate in
adjacent stellar layers. It follows that electron capture (which includes
neutronization) inside the gain radius (figure 9) in the cooling region and
neutrino absorption in the heating region, play a key role in SNe.

Nucleon based interactions such as the modified URCA processes
[46] also contribute to the production, scattering and absorption of neutrinos
in the cooling and heating regions. Other particle processes which play a role
in these regions are pair annihilation, plasmon decay, the Photoneutrino process
and Bremsstrahlung. These processes are shown in tables 6 and 7 respectively:

The processes listed in tables 5, 6 and 7 hide the importance of
each reaction type and types of neutrinos produced. For instance there are
electron, muon and tau neutrinos created, however the electron-neutrino flavour
dominates in these nuclear reactions as more of them are created. Additionally
the most prevalent neutrino production processes in SNe are Bremsstrahlung, the
Photoneutrino process and those related to electron capture (including
neutronization) and absorption [45], as these are dependent on the high
temperatures and densities found around SNe core and shock fronts. There are
also neutrino interactions which involve neutrino scattering in the heating and
cooling regions of SNe as shown in table 8:

A summary of these processes is provided in table 9. It is
estimated that 10^53 ergs of energy [47] are released in a SNe explosions hence
important neutrino production and absorption processes (highlighted below) play
a key role in this vast amount of energy produced:

Back to Top
|
Back to
High Mass Stellar Evolution
Conclusion
Our understanding of SNe physics such as explosive
nucleosynthesis and neutrino processes and our ability to exploit single and
multidimensional modelling techniques to reproduce these effects is maturing
rapidly. It is clear now that neutrino physics plays a key role in producing and
sustaining SNe explosions and that explosive nucleosynthesis is responsible for
the production of various elements found in the interstellar medium. It is also
becoming clear that processes such as neutron capture as part of explosive
nucleosynthesis, beta decay and neutrino physics are bridging the gap between
theory and observations.
Although multidimensional chemical yields, energetics and
delayed explosions are only partially solved, 3-d SNe models hold the key to
required improvements in results relating to rotation, convection and ejecta
asymmetries which correlate with results of observed light curves and derived
chemical and mass yields. Further improvements in microphysics being groomed in
1-d models is expected to be used to complement multidimensional models so to
provide a holistic view of the SNe core collapse processes. In addition further
observations, analysis and results will continue to provide constraints for
theoretical models and this will assist in further bridging the gap between
theory and observations.
Back to Top
|
Back to
High Mass Stellar Evolution
References:
[1] Fryer C. L., Heger A., 1999,
Core-Collapse Simulations
of Rotating stars, http://arxiv.org/abs/astro-ph/9907433
[2] Livne E., Burrows A., Walder R., Lichtenstadt I., Thompson
T. A., 2003,
Two-Dimensional, Time-Dependent, Multi-Group, Multi-Angle Radiation
Hydrodynamics Test Simulation in the Core-Collapse Supernova Context, http://arxiv.org/abs/astro-ph/0312633
[3] Arnett D., Bazan G., 1997,
Two-Dimensional
Hydrodynamics of Pre-Core Collapse: Oxygen Shell Burning http://arxiv.org/abs/astro-ph/9702239
[4] Kozma C., Fransson C., Hillebrandt W., Travaglio C.,
Sollerman J., Reinecke M., Ropke F. K., Spyromilio J., 2005,
Three-Dimensional Modelling
of Type Ia Supernovae – The Power of Late Time Spectra, http://arxiv.org/abs/astro-ph/0504317
[5] Reinecke M., Hillebrandt W., Niemeyer J. C., 2002,
Three-Dimensional
Simulations of Type Ia Supernovae, http://arxiv.org/abs/astro-ph/0206459
[6] Fryer C. L., Warren M. S., 2003,
The Collapse of Rotating
Massive Stars in 3-Dimensions, http://arxiv.org/abs/astro-ph/0309539
[7] Janka H.-Th., Buras R., Kifonidis K., Marek A., Rampp M.,
2004, Core-Collapse
Supernovae at the Threshold, http://arxiv.org/abs/astro-ph/0401461
[8] Ropke F. K., 2004,
Following Multi-Dimensional
Type Ia Supernova Explosion Models to Homologous Expansion, http://arxiv.org/abs/astro-ph/0408296
[9] Hungerford A. L., Fryer C. L., Warren M. S., 2003,
Gamma Ray Lines From
Asymmetric Supernovae, http://arxiv.org/abs/astro-ph/0301120
[10] Fryer C. L., Warren M. S., 2002,
Modelling Core-Collapse
Supernovae in 3-Dimensions, http://arxiv.org/abs/astro-ph/0206017
[11] Travaglio C., Kifonidis K., Muller E., 2003,
Multi-Dimensional
Nucleosynthesis Calculations of Type II SNe, http://arxiv.org/abs/astro-ph/0305440
[12] Travaglio C., Hillebrandt W., Reinecke M., Thielemann
F.-K., 2004,
Nucleosynthesis in Multi-Dimensional SN Ia Explosions, http://arxiv.org/abs/astro-ph/0406281
[13] Reinecke M., Hillebrandt W., Niemeyer J. C., 2001, Refined Numerical Models
for Multidimensional Type Ia Supernova Simulations, http://arxiv.org/abs/astro-ph/0111475
[14] Burrows A., Walder R., Ott C. D., Livne E., 2004, Rotating Core Collapse and
Bipolar Supernova Explosions,
http://arxiv.org/abs/astro-ph/0409035
[15] Hillebrandt W., 2004, Supernova Explosion Models:
Predictions Versus Observations,
New Astronomy Reviews
48 (2004) 615-621
[16] Mezzacappa A., 2000, Supernova Studies at ORLaND,http://arxiv/.org/abs/astro-ph/0010577
[17] Burrows A., Thompson T. A., 2002, The Mechanism of
Core-Collapse Supernova Explosions: A Status Report, http://arxiv.org/abs/astro-ph/0210212
[18] Janka H.-Th., Buras R., Rampp M., 2002, The Mechanism of
Core-Collapse Supernovae and the Ejection of Heavy Elements,
http://arxiv.org/abs/astro-ph/0212317
[19] Hillebrandt W., Niemeyer J. C., Reinecke M., Travaglio C.,
2003, The Physics
and Astrophysics of Type Ia Supernovae Explosions,
Memorie della Societa’
Astronomica Italiana, Vol. 74, 942
[20] Badenes C., Bravo E., Borkowski K. J., 2003, Thermonuclear Supernova
Explosions and Their Remnants: The Case of Tycho,
http://arxiv.org/abs/astro-ph/0309013
[21] Burrows A., Ott C. D., Meakin C., 2003, Topics in Core-Collapse
Supernova Theory,
http://arxiv.org/abs/astro-ph/0309684
[22] Blinnikov S., Sorokina E., 2004 , Type Ia Supernova Models:
Latest Developments,
Astrophysics and
Space Science 290: 13-28
[23] Liebendoerfer M., Mezzacappa A., F.-K. Thielemann, Messer
O. E. B., Hix W. R., Bruenn S. W., 2001, Simulation of the
Spherically Symmetric Stellar Core Collapse, Bounce, and Postbounce Evolution of
a 13 Solar Mass Star with Boltzmann Neutrino Transport, and Its Implications for
the Supernova Mechanism,
http://arxiv.org/abs/astro-ph/0005366
[24] Liebendoerfer M., Mezzacappa A., Thielemann F.-K., 2001,
Conservative General
Relativistic Radiation Hydrodynamics in Spherical Symmetry and Comoving
Coordinates,
http://arxiv.org/abs/astro-ph/0012201
[25] Rampp M., Janka H. T., 2002, Radiation hydrodynamics
with neutrinos: Variable Eddington factor method for core-collapse supernova
simulations,
http://arxiv.org/abs/astro-ph/0203101
[26] Rampp M., Janka H. T., 2000, Spherically Symmetric
Simulation with Boltzmann Neutrino Transport of Core Collapse and Post-Bounce
Evolution of a 15 Solar Mass Star,
http://arxiv.org/abs/astro-ph/0005438
[27] Fleurot F., 1998, Evolution of Massive Stars,http://nu.phys.laurentian.ca/~fleurot/evolution/evolution.php
[28] Burrows A., Hayes J., Fryxell B., 1995, On the Nature of Core
Collapse Supernovae Explosions,
http://arxiv.org/abs/astro-ph/9506061
[29] Herant M., Benz W., Hix W. R., Fryer C. F., Colgate S.,
1994, Inside the
Supernova: A Powerful Convective Engine,
http://arxiv.org/abs/astro-ph/9404024
[30] Colgate S. A., White R. H., 1966, Astrophysics Journal 143,
626
[31] Arnett W. D., 1967, Canadian Journal of Physics 45, 1621
[32] Wilson J. R., Mayle R., 1988, Physics Report 163 63
[33] Wilson J. R., Mayle R., 1993, Physics Report 227 97
[34] Mayle R., Tavani M., Wilson J. R., 1993, Astrophysics
Journal 418 398
[35] Totani T., Sato K., Dalhed H. E., Wilson J. R., 1993,
Astrophysics Journal 496 216
[36] Kotake K., Yamada S., Sato K., 2004, North-South Neutrino
Heating Asymmetry in Strongly Magnetised and Rotating Stellar Cores
http://arxiv.org/abs/astro-ph/0409244
[37] Wang L. Baade D., Hoeflich P., Khokhlov A., Wheeler C.,
Kasen D., Nugent P. E., Perlmutter S., Fransson C., Lundqvist P., 2003, ApJ, vol
591, p1110
[38] Gibson B., 2005, Stellar Astrophysics - The Physics of
Supernovae, Swinburne Astronomy Online
[39] Hoflich P., Wheeler J. C., Thielemann F. K., 1997, Type Ia SNe: Influence of
the Initial Composition on the Nucleosynthesis, Light Curves, Spectra and
Consequences for the Determination of Ωm
& Δ, http://au.arxiv.org/archive/astro-ph/9709223
[40] Washington State University, 1998, Nucleosynthesis in
Supernovae, http://www.int.washington.edu/PHYS554/chapter5_98.pdf
[41] Bethe H., 1993, AJ Vol. 412, Part 1, no. 1
Abstract available from:
http://www.csa.com/partners/viewrecord.php?requester=gs&collection=TRD&recid=A 9346316AH&q=%22gain+radius%22&uid=1053751&setcookie=yes (Click
Here)
[42] Woosley S. E., Wilson J. R. Mathews G. J., Hoffman R. D.,
Meyer B. S., 1994, ApJ 433 229W
Abstract available from:
http://adsabs.harvard.edu/cgi-bin/nphbib_query?bibcode=1994ApJ...433..229W&db_key=AST
[43] Periodic Table of Elements,
http://www.webelements.com/
[44] Gagnon S., Beta Decay,
http://education.jlab.org/glossary/betadecay.html
[45] Thompson T. A., Burrows A., Horvath J. E., Thermalization and
Production of Neutrinos in Dense Nuclear Matter,
http://zenith.as.arizona.edu/~burrows/eos.wind.thermal/thermal.html
[46] Leung G. Y. C., Dense Matter Physics, University of
Massachusetts Dartmouth,
http://www.cis.umassd.edu/~gleung/physicsfo/Dense%20Matter/Dense%20Matter%20Physics%206.htm
[47] Fleurot F., 1998, Type-II Supernova Neutrinos
http://nu.phys.laurentian.ca/~fleurot/supernova/supernova.php
[48] Woosley S. E., 2004, Neutrino Powered Explosions, Fall Back
and Mixing,
http://www.ucolick.org/~woosley/ay220cnotes.html
[49] Gibson B., 2005, (private communication)
Back to Top
|
Back to
High Mass Stellar Evolution
Credits and Comments:
Cover Image:
Courtesy Russel S.,
Halls B., 2004, “Exploring Stars & The Milky Way - Supernovae”, Swinburne
Astronomy Online
Figure 1:
Adapted from Akgun T.
R., “Shock Revival Through Neutrino Heating in Supernovae”, Department of
Astronomy and Space Sciences – Cornell University.
Figure 2:
Courtesy Woosley S.
E, “Neutrino Powered Explosions, Fall Back and Mixing”, and Mezzacappa et al.,
1998, ApJ 495, 911.
This figure depicts a “standard” 15 solar mass progenitor in a 1-d simulation
running for 500 milliseconds using a flux limited multi-group neutrino transport
coupled to a 2-d hydrodynamical code.
Figure 3:
Courtesy
Kotake K., Yamada S.,
Sato K., 2004, “North-South Neutrino Heating Asymmetry in Strongly Magnetised
and Rotating Stellar Cores”
This figure depicts SNe
explosions with varying amounts of angular momentum and magnetic field strength.
The colour coding indicates the level of entropy in the system.
Figure 4: Courtesy Travaglio
C., Hillebrandt W., Reinecke M., Thielemann F.-K., 2004, “Nucleosynthesis in
Multi-Dimensional SN Ia Explosions”
Front evolution of centrally
ignited and multi-point ignited (floating bubble) models
Figure 5:
Courtesy Gibson B.,
2005, “Stellar Astrophysics - Chemical Evolution”, Swinburne Astronomy Online
Figure 6:
Courtesy Russel S.,
Halls B., 2004, “Exploring Stars & The Milky Way - Supernovae”, Swinburne
Astronomy Online
Figure 7:
Courtesy Russel S.,
Halls B., 2004, “Exploring Stars & The Milky Way - Supernovae”, Swinburne
Astronomy Online
Figure 8:
Courtesy from
Hillebrandt W., Niemeyer J. C., Reinecke M., Travaglio C., 2003, “The Physics
and Astrophysics of Type Ia Supernovae Explosions”, Memorie della Societa’
Astronomica Italiana, Vol. 74, 942
Synthetic UBVI light curves
predicted by a centrally ignited 3-d model compared to observational data from
normal and subluminous SNe.
Figure 9:
Courtesy Mackie G.,
Fluke C., 2005, “Stellar Astrophysics – Evolving Theories”, Swinburne Astronomy
Online
Table 1:
Adapted from “The
Physics of Supernovae”, Swinburne Astronomy Online
Table 2: Adapted from “What
Powers the Stars?” Swinburne Astronomy Online
Table 3: Adapted from
“Reaction Rates”, Swinburne Astronomy Online
Table 4: Adapted from “The
Physics of Supernovae”, Swinburne Astronomy Online
Back to Top
|
Back to
High Mass Stellar Evolution
1 The Term SNe in this context may be
used interchangeably to refer to “Supernova” and “Supernovae” respectively
2 Progenitor stars are stars
simulated in stellar models of core collapse SNe.
3 Anisotropy is used to describe a
condition which exhibits different properties in different directions
4 Deflagration occurs when
thermonuclear burning travels outwards from the stellar core at a speed less
than the speed of sound. Detonation occurs when thermonuclear burning travels
outwards from the stellar core at a speed greater than the speed of sound
5 From this point “neutrino” may be
used to indicate either ‘neutrino’ or ‘antineutrino’.
6 SNe processes involve explosive
nucleosynthesis, neutron capture (s and r-processes) and neutrino processes.
7 “Particle” in this context will
generally be used to refer to leptons (electron, muon, tau, electron neutrino,
muon neutrino, tau neutrino and their corresponding antiparticles)
8 “Nucleons” in this context will
generally be used to refer to hadrons such as protons neutrons
9 This process is also referred to as
de-leptonization
10 This is different to Type Ia SNe,
as Type Ia’s don’t posses the extensive layered structure of heavier SNe
progenitors.
Back to Top
|
Back to
High Mass Stellar Evolution |

