Back to Exoplanets

Introduction
The Solar Nebula Hypothesis and the Core Accretion Model
Exoplanets
Radial Velocity
The Transit Method
Types of Systems
Exoplanetary System Formation
Core Accretion Model Revisited
Earth-like Planets
Summary
References
Image Credits

Dynamics of Extrasolar Planetary Systems 

Introduction: 

There are two competing theories of planet formation in a system of planets: the Core Accretion Model and the Disk Instability Model (sometimes called the Gravity Instability Model). Because we are able to study our own Solar System, we are reasonably certain the formation of it occurred via the Solar Nebula Hypothesis with the Core Accretion Model responsible for planet formation. The cores of the planets, the delineation between terrestrial and gas giant planets and the ingredients of chondrites serve as evidence to support this theory; however, the discovery of numerous exoplanetary system have some astronomers favoring the Disk Instability Model over the Core Accretion Model. Could this be due to massive planets forming close to their host star or due to inward migration of Jupiter-sized planets? Could the sampling of large planets be the result of instrument sensitivity? While it is difficult to create a unified theory of solar system formation, it may be possible that planetary systems form with a combination of both theories. In addition to discussing planetary formation, I will also introduce the methods in detecting extrasolar planets and the possibility of detecting Earth-like planets. The advantages and disadvantages of detection methods are also covered as this can also give important information to the known dynamics of extrasolar planetary systems. A note to the reader: the terms exoplanet and extrasolar planet are used interchangeably.

Back to Top

The Solar Nebula Hypothesis and the Core Accretion Model: 

To understand planetary systems (also known as exoplanetary systems), we must first understand our own system of planets. This may sound simple, but we are still learning about our Solar System – however, there are some things we do know. For example, we are reasonably certain that our own Sun and the planets that orbit the Sun formed from a cloud of gas and dust – or molecular cloud. This is the basis of the Solar Nebula Hypothesis – that from a cloud of dust and gas, our Solar System formed to what we see today: a Sun, four rocky terrestrial planets, an asteroid belt, four “gas-giant” planets, Pluto and the other Kuiper Belt Objects, and the Oort cloud (and the variety of debris within).

(Image Credit)

While many pages can be written to describe the process of our Solar System formation, the basic steps are as follows[1] (Freedman and Kaufmann, 2005):

• Within the arms of our Milky Way Galaxy exist clumps of dust clouds that contain molecular hydrogen and dust.
• Some mechanism causes one of these clouds to collapse – perhaps a nearby supernova or spiraling density waves within the arms of the galaxy.
• As the cloud collapses, it begins to rotate and flatten out.
• Gravity and pressure begin to increase their influence – gravity wants to collapse the cloud while pressure tries to balance the force to prevent collapse.
• Eventually gravity wins (in this case) – pressure is exerted on the center of the cloud and by the Kelvin-Helmholtz contraction the center of the cloud heats up.
• Continual heating and cloud spinning results in a central proto-star – that is a star that has not begun the fusion process – forms while dust in the newly formed disk begins to coalesce.
• The proto-star continues to gain mass, with increasing heat and pressure while planetesimals form with the accreted dust particles.
• Continued accretion of material form even larger planetesimals while the heat from the proto-star cause the ices in the inner portion of the disk to melt away – the line that separates the temperature differential between the inner and outer disk is called the “snow-line.”
• Terrestrial protoplanets form within the inner disk. The same occurs in the outer disk but these protoplanets have more gaseous material available to collect huge atmospheres. The planetesimals differentiate – that is due to heat, pressure and added mass, heavier elements sink towards the core.
• At this time, the proto-star gets enough mass and pressure to initiate fusion – the star ignites and a T Tauri wind blows away any non-accreted material within the disk.
• What remains are a newly formed star and a system of planets.

The collection of dust particles to form planetesimals to protoplanets to full fledged planets is the crux of the Core Accretion model. Primitive refractory material has been found in Chondrites that support this theory (Beatty et al., 1999). The fact that planets have cores – which formed by differentiation – also support this theory. 

There are two issues with the Core Accretion model, and they have to do with the gas giant planets (Jupiter-like planets). Given the time it takes to form a terrestrial planet prior to the T Tauri phase, it is believed there was not enough time to form the outer massive planets. In addition, the mass of Jupiter would have prevented the formation of Uranus and Neptune in their current positions. The Disk Instability Models explains how such large planets can form so rapidly – and given that formation occurs so fast, the all the material is available for planet formation (i.e. Uranus and Neptune) prior to the T Tauri Phase. In other words, the gas giant planets formed directly from the solar nebula (Freedman and Kaufmann, 2005) and not by accretion. For the large planets to form, the material accretes by a process called “runaway accretion” – a process that does not allow time enough for a core to form[2]. However, there are four major flaws with the Disk Instability Model: 

• This model does not explain the cores of Jupiter, Saturn, Uranus and Neptune – each of these planets have a core even though the model suggests they should not have one.
• If Jupiter (being the largest, most massive planet in or Solar System) formed directly from the solar nebula, it should have the same composition as the Sun – it does not.
• The Disk Instability Model should have formed much larger gas giant planets.
• This model does not explain why the terrestrial planets exist.

These issues are still being worked out – and with the data collected regarding exoplanetary systems it is hoped that the issue of Core Accretion versus Disk Instability will be worked out. 

Planetary formation via the Solar Nebula Hypothesis has been demonstrated by three phenomena: 

• Proplyds (proto-planetary disks) discovered in the Orion Nebula
• Herbig-Haro Objects
• Image of individual orbital rings around Beta Pictoris  

Proplyds[3] – shown on the left – are a system of planets in the making. Much of the material here has been “blown away” by the surrounding hot O and B type stars, which is why the T Tauri star is visible. The dense region surrounding the T Tauri star is the circumstellar disk that has accreted to a disk shape. Planets will form here (we are actually uncertain if planets will form within these proplyds as there may not be enough material).   More information on this image and the Proplyds can be found on the Hubble Space Telescope website.

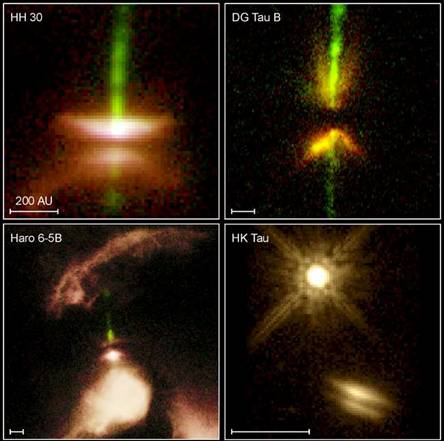
Herbig-Haro objects are a T Tauri star (or proto-star) surrounded by the “correct” amount of material that emits visible jets (Freedman and Kaufmann, 2005). In other words, there is enough disk material to stimulate continued system evolution with the presence of the jets – seen here 90 degrees from the plane of the disk - while the T Tauri Star itself is not visible.   According to the Hubble Space Telescope website, the action and morphology of these disks demonstrates the circumstellar disk material is still falling onto the proto-star.

(Image Credit)

The most dramatic image of a solar system in progress is the circumstellar orbits image around Beta Pictoris. This image appears to demonstrate the sweeping up of debris to accrete planets - a good example of the Core Accretion Model.

Back to Top

Exoplanets: 

Images of Proplyds, Herbig-Haro objects and circumstellar disks suggest our theory on the formation of our Solar System by the Solar Nebula Hypothesis is correct, but it says nothing on how the planets formed (although the Beta Pictoris image does demonstrate possible accretion). In fact, these three remarkable images show no planets. 

The only image we have of an extrasolar planet is the remarkable image from the European Southern Observatory. As the caption in the image states – it is the planetary companion to Brown Dwarf 2M1207. The distance of the planet is twice as far as the Sun-Neptune distance and was imaged using a variety of infrared wavelengths. More detail is available on the ESO Press Release 12/05 webpage. 

(Image Credit)

Exoplanet research is one of the hottest new fields in Astronomy – and its one that both amateurs and professionals can participate. These images are very dramatic and prove all of the efforts of the various projects that search for these planets, however imaging a random star will not guarantee an image of a planet. We need to know where to look, and this is where detection takes place.

There are two main methods of detection with a third and fourth method gaining momentum. 

• Radial Velocity
• The Transit Method
• Astrometry
• Microlensing

Radial Velocity and the Transit Method are, at the moment, the primary methods of detection. Astrometry is also used to detect planets, but this method is most useful in long term follow up of known exoplanetary systems – it also detects a wobble (discussed in the radial velocity section below) but without using a spectrometer - instead, it uses lots of math. In addition, the number of planets detected with the astrometric method is very low, and accuracy  is at best equal to Doppler measurements (Sozzetti, 2005). Astrometry does have its strong points. For one, the mass of the detected planet is measured accurately compared to the radial velocity method. In addition, planets with larger orbits can be detected, but this can take years – even decades – to complete a study. 

Microlensing also deserves a brief introduction, but only a handful of planets have been detected using this method. At best, microlensing serves more as a survey to determine how many Earth-sized planets there are. The distances to these stars – at least the distance to allow for microlensing events – are too far away for continued study (Lunine, 2005). How microlensing works is by detecting a gravity lens, however a star is not capable of bending the image of another star (like those wonderful galaxy lenses seen in Hubble images) – due to its low mass relative to galaxies. Instead, the brightness of the star increases a bit. If the lensing star has a planet, the brightness of the lensed star will increase a bit more for a short period of time. It is safe to say that not much information about the planet can be gleaned from this data – only that a planet is present.

Back to Top

Radial Velocity 

The first exoplanets were discovered by what is called the “wobble.” This sounds low tech, but this is very significant (the “wobble” is also associated with astrometric detection). When two objects orbiting each other contain any mass, they will have an affect on each other resulting in an inertial center - called the barycenter (Mayor and Frei, 2003). Using our own solar system as an example, Saturn and Jupiter have enough mass that the effect is a wobble of the Sun (Marcy and Butler, 1997). The net affect of both planets produce a wobble of around 13 m/s and if Jupiter were the only planet, a wobble of around 12 m/s would be present.

A spectrometer detects shifting in the absorption lines of the stars spectra. This shift can be measured using simple mathematics that compares a reference object containing identical spectra to the shifted object. With this information, it is possible to determine the velocity of the object. 

Equation 1

Determining Doppler redshift and blue shift:

z = =

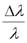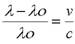
z = redshift

∆λ = shift in wavelength

λ = wavelength of stationary object

λο = stationary wavelength – reference spectra

v = velocity

c = speed of light (300,000 km/s)

It is important to state that:

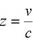
Equation 1 is the basis of determining the orbital velocity of the object orbiting the affected star or determining the radial velocity of the affected star. Once the orbital velocity is determined, simple usage of Kepler’s Third Law will determine the distance the planet is to the host star. 

Kepler’s Third Law: 

P² = a³

P = object’s rotation period in years

a = object’s distance to star in Astronomical Units
 

By using a high resolution spectrometer, standard Doppler measurements are around 15 m/s; however, a method of introducing iodine gas near the slit entrance has allowed for precision measurements of up to 3 m/s (Butler et al, 1996).  The iodine is used to create a composite spectrum to overlay the spectra of the analyzed star and enhances our view of the absorption lines while acting like a ruler. By eliminating any uncertainty between stellar absorption lines with a laboratory standard, precision measurements are attained.  

There are some disadvantages to using the Doppler shift to measure radial velocities: the star must be as close to the host star are possible. For example, one of the first exoplanets discovered – the companion to 51 Pegasi – is only 0.05 AU’s[4] (Mayor and Frei, 2003). That means the planet, which is 0.5 times the mass of Jupiter, is much closer than the orbit of Mercury is about our Sun. For planets that orbit at a larger distance from the star, more precise astrometry measurements are desired – mostly because larger orbits require many years to study versus days of a closer orbiting planet. In addition, the mass of the planet is determined by m sin i - which varies based on the angle of the system relative to the observer (m = mass, i - inclination). If the system is face on (God's eye view), the determination of mass is not accurate at all; however, if a system is closer to an edge on view, the determination of mass is more accurate. This is often called the "m sin i problem."

Back to Top

The Transit Method 

Another important method of detection is by measuring the transit of a planet over the face of the host star. By performing careful photometric plots of the host star, drops in stellar brightness as a planet moves across the face of the star can be measured. The first ever measurement of a stellar transit was made by the Elodie group (discoverers of the companion to 51 Pegasi) and the David Latham group (Mayor and Frei, 2003) - also known as project STARE.  Currently this method is used to study known exoplanetary systems. With the m sin i problem discussed above, the transit detection allows astronomers to determine the value for i in order to determine planet mass more accurately. Once the mass is determined by Doppler, diameter of the planet can clue us in to density and even infer an atmosphere.

The transit method can only be used for a planetary system that is directly in our line of sight. An additional limitation is the size of the planet. A planet 0.64 times the mass of Jupiter will decrease the brightness of its host star by about 0.0011 magnitudes – this is a small change in brightness (Mayor and Frei, 2003). Determining the transit of an exoplanet is not as difficult as performing radial velocity measurements. While determining the radial velocity requires carefully calibrated equipment, specialized spectroscopes, and lots of patience, determining the transit only requires the skill of photometry, a telescope and CCD camera, and a personal computer. Quite simply, photometry is the study of stellar brightness. While there is a danger that a transiting planet can be confused by other phenomenon like variable stars, proper measurements will rule this out as variable stars and transiting binary stars have specific patterns of photometric changes (Butler, 1998).  

Back to Top

Types of Systems 

The majority of exoplanets discovered have been around metal rich main sequence stars (with a few exceptions) – specifically stars with a spectral class of F, G and H (Butler et al, 2000). The reasons are twofold: 

1. F, G, and K type stars are “normal” sized stars like our Sun, and will more than likely exist long enough for planets to form. Larger, hotter burning stars end their lives much sooner so the possibility of mature planets to form is highly unlikely; although planets have been found to orbit stellar remnants such as pulsars.
2. Metal rich stars contain heavier elements in their atmospheres as a result of enriched molecular clouds from which they have formed. This results in more absorption lines to be examined.

It is much more likely that a planet capable of having a substantial atmosphere will require a metal-rich host because this will require silicate and carbonate materials - material from a metal-rich molecular cloud from which the system formed. 

This image, from the Extrasolar Planet Encyclopedia website, demonstrates the metallicities and masses of the stars hosting planets thus far. This coincides with the data from Butler et al., 2000. 

Back to Top

Exoplanetary System Formation: 

As of April 16, 2006 there are 188 detected exoplanets. The vast majority (176 of them) have been detected using the Radial Velocity method. 4 planets have been detected by direct imaging, 4 planets orbiting pulsars have been detected and 4 planets detected by microlensing (http://vo.obspm.fr/exoplanetes/encyclo/catalog.php).  Since the much publicized discovery of 51 Peg companion in 1995, the field of exoplanetary study has grown rapidly. For the initial years of exoplanet detection, spectrometer resolution (for the radial velocity searches) was limited to a 13 m/s detection capability. With this level of sensitivity, the majority of planets discovered were massive planets very near the host star. By using a technique of introducing an iodine gas into a spectrometer, it was found that the spectral resolution increased to an incredible 3 m/s (Butler et al., 1996).  

Since the introduction of the higher resolution spectrometer, more planets have been discovered but with the same parameters as the earlier planets – high mass “hot-Jupiters” orbiting very near the host star. The number of these high-mass, close orbit planets was initially thought to be a sampling issue, but higher resolution spectroscopy is detecting more of these high mass planets. There are two possible explanations as to why these bodies are so close to their host: either they formed close to the host star, or they migrated inward. In both cases, the Core Accretion Model does not apply (Boss, 2000).  

Transiting planets, like HD 209458b, can have a significant atmosphere (Arribas et al., 2006). This is thought to be the result of the planet being so close to the host star; the planet “swells up” to appear much larger than it would had the planet been allowed to condense. This could be one reason why there are so many Jupiter-sized bodies in such tight orbits. This type of planet, so easily “puffed up,” coincides with formation via Disk Instability as there is no definitive core keeping the planet together. 

Two major issues confront astronomers when studying exoplanetary systems: 

• Prevalence of high-mass planets in close orbits
• Highly eccentric orbits

As briefly mentioned, the presence of high-mass planets in close proximity to the host star does not fit the Core Accretion Model. For such a high mass planet to form so rapidly requires immediate formation – the crux of the Disk Instability Model. Migration of the newly-formed planet also requires a proto-planetary disk of higher density. This provides the material for formation as well as the “drag” required to cause the planet to migrate (Boss, 2000) - which is believed to be the primary reason for the existence of tight orbit, high mass planets. Planets are not believed to form close to the star as the star itself would grab up any debris that would have created the planet.

While simulations by Boss, 2000 indicate Disk Instability can occur with a proto-planetary nebula of 0.1 solar mass (believed to be the mass of our own solar nebula prior to Solar System formation), Goldreich et al., 2004 states the disk material required for Disk Instability to work must be optically thick (thicker than our own Solar Nebula). In addition, disk clean-up (the material not accreted to create planets) is a poorly understood process. Any significant debris left in the proto-planetary disk could provide the necessary drag to force planetary migration – especially if there are few bodies on the system. 

The high eccentricity of many discovered exoplanets caused some concern for the Core Accretion Model as this feature of exoplanetary systems seems to offer additional evidence to the Disk Instability Model – the sudden creation of a planet that migrated as a result of disk debris and interactions with other rapidly accreting bodies. However, simulations by Innanen et al, 1998 of our own Solar System demonstrate that without the Earth-Moon system, the inner planets – Mercury, Venus and Mars - would evolve into highly elliptical orbits. In some simulations, Mercury was completely ejected. This results in an important (and new) system dynamic called orbital resonance - a key part in planetary system stability. In a project I did for a previous class, I determined the stability component of the inner planet of the Gliese 876 system to the other two massive planets. The result is that the eccentric orbits of Gliese 876b (1.9 Mjup) and Gliese 876c (0.6 Mjup) are the results of interactions with each other and Gliese 876d (0.023 Mjup) is the stabilizing factor that keeps the eccentric orbits stable (Murphy, 2005). This inner planet has also prevented further migration of the larger bodies. 

Back to Top

Core Accretion Model Revisited: 

The Disk Instability Model does present some compelling data in the present condition of exoplanetary systems, but even now smaller planets are being discovered in several of these existing systems. This can be attributed to better, more sensitive equipment and continued study and simulations of current systems. Some very interesting data plots are available from the Extrasolar Planets Encyclopedia website. For example, the following plots show trends of planet mass versus discovery date, planet count by discovery date, semi-major axis (S.M.A.) versus discovery date and S.M.A. count by discovery date.  

These images show that as time reaches the present, we are able to detect more lower-mass planets at greater orbits as well as more balanced overall system. With orbital resonance as a factor, this greatly affects the overall system evolution when considering Disk Instability.

These plots demonstrate that smaller planets as well as larger orbits are being discovered. These are certain to provide the necessary data to help re-introduce the Core Accretion Model as far as exoplanetary formation. The reasons are: 

• Larger planets found at greater distance
• Increased number of planets to explain orbital resonance and ecliptic orbits

Only with the collection of more data can we solidify either theory, or as Boss, 2000 suggests: the formation of planetary systems could be the marriage of both the Core Accretion Model for terrestrial, high density planets and the Disk Instability Model for gaseous, lower density planets. But it's clear that the bias towards high mass planets is due to current detection methods.

Back to Top

Earth-like Planets: 

Detecting an Earth-like planet is the absolute Holy Grail of exoplanet research. What is exciting is that observations are well under way that will pave the way for this detection. Currently, the favored mechanism for detecting an atmosphere is using spectroscopy coupled with a transit of the planet around the host star. The methodology is rather simple: with the spectra of the star identified, simply subtract this known spectrum with the spectra of the planet. Any gaseous atmosphere will be detected. This sounds rather simple and in a way it is with the only limitation being equipment sensitivity.  

Using a technique called Integral Field Spectroscopy (which is a combination of high resolution spectroscopy and wide field detection spectroscopy), sodium was detected in the atmosphere is planet HD 209458b (Arribas et al., 2006). This technique is planned to be used in the future DARWIN and TPF missions, but the detection of sodium using a terrestrial based telescope demonstrates that results can also be shown with the Hubble Space Telescope or the future James Webb Space Telescope. 

One experiment that was set to detect and explore Earth-like worlds was NASA’s Terrestrial Planet Finder (TPF). Using a similar layout to DARWIN, this would have been able to detect Earth-like atmospheres. Unfortunately, budget cuts have forced NASA to send the TPF to the guillotine (McDowell, 2006). However, lets consider the search as if the TPF were still in the wings; what would the TPF look for? The spectra of the atmosphere would need to be captured; however, the difficulty is in the optical wavelengths – that is contamination by the host star. To get around this, the spectra would be captured in the infrared.

(Image Credit)

In the infrared, water and ozone will be more prominent (Lunine, 2005).  Since water is required for life, the presence of water in the spectrum of the detected planet is a good indicator that life is possible. The presence of Ozone will demonstrate than some biological process is occurring as oxygenation of the atmosphere can occur through natural biological processes.

The drawback is that the spectrometer on the TPF will be unable to detect liquid water – which is required for life to evolve. Water detected in an atmosphere does not mean that liquid water exists on the surface – but the detection of any water in an atmosphere of an extrasolar planet is significant.
                                     
While the TPF project has been scrapped, the DARWIN is still underway. With a predicted launch date of 2015, the DARWIN will contain four free-flying 3-meter infrared telescopes. The purpose is to be a nulling interferometer to directly detect and image planets by “nulling out” the host star. Because the telescope operates in the infrared, the orbit of the spacecraft will be at L2 with a distance of 1.5 million kilometers opposite the Sun in order to shield the telescope from the “heat” of the Earth and Sun (DARWIN). 

While direct detection of Earth-sized bodies as well as detection of an Earth-like atmosphere is no guarantee we will find life, it does provide us with definitive proof that Earth-like bodies do exist in the Universe. This certainly does have some impact on the social and religious environments on Earth, but that is another story! 

Back to Top

Summary: 

There is a lot of information here that covers a broad spectrum of the exoplanet phenomenon. To recap, exoplanets have been discovered using the venerable radial velocity method. While this method has discovered the most planets – and will not doubt continue to do so – the transit method and astrometry method are better at nailing down the actual mass of the discovered planet, supplementing important data to support the radial velocity measurements; however, currently the radial velocity is biased towards high massed planets due to its current sensitivity. The transit method is unfortunately limited to close orbiting bodies of high mass that are directly in our line of site while astrometry is limited to planets with larger orbits and therefore require long bits of time to observe. Microlensing is a method to give a survey of Earth-sized worlds but cannot help with any additional data beyond detection. Direct detection has not been covered with the exception of the first ever detection from the ESO. The reason is not only space constraints on this paper, but there are only 4 planets credited to direct detection.

The future of direct detection is very interesting indeed, and one project deserves mention: PLANETPOL (http://www.ing.iac.es/PR/wht_info/whtplanetpol.html). While in its infant stages, the goal is to directly detect planets by detecting the polarized light reflected by the planet emanating from the host star (which is not polarized).

While we are reasonable certain of our own Solar System formation through the Core Accretion Model, the discovery of exoplanets with high masses in close orbits along with high elliptical orbits have the Disk Instability Model preferred in the formation of these systems. While this has some ramifications on our Core Accretion Model theory, the issue may simply be sampling as more sensitive instrumentation are finding smaller bodies. In addition, the result of ellipsis could also be interactions with other planets – simply a consequence of planetary system formation. Planetary migration due to the density of the protoplanetary disk may also play a role in the presence of the “hot-Jupiters.” It is possible that planet formation is a combination of the Core Accretion and Disk Instability Models. 

Detection of an atmosphere around a high-mass planet has been detected, but detection of an Earth-like atmosphere – as well as directly detecting an Earth-sized planet – will require infrared detection with a space-based telescope. The DARWIN mission will provide the necessary resources to detect these bodies as well as look for the tell-tale sign of a life bearing atmosphere. 

Exoplanet research is an exciting field in Astronomy. Since 1995 when the first planet was detected, the field shows no sign of slowing down. While the models of exoplanetary solar systems seem to fit the Disk Instability Model – an unfavorable model of planet formation – further data collection seems to point to a combined Core Accretion Model and Disk Instability Model in the creation of a planetary system. It is hoped that missions like DARWIN as well as continued follow-up using methods like astrometry and transit studies will provide the data to solidify these theories. 

Back to Top

References: 

Arribas, Santiago et al. “Exploring the Potential of Integral Field Spectroscopy for Observing Extrasolar Planet Transits: Ground-based Observations of the Atmospheric Na in HD 209458b.” PASP, 118:21-36. January 2006. 

Beatty, Kelly J.; Petersen, Carolyn C. and Andrew Chaikin. The New Solar System. Fourth Edition. Cambridge University Press, 1999. 

Bedding, Thomas et al. “Evidence for Solar-Like Oscillations in ß Hydri.” The Astrophysical Journal, 549: L105-L108, March 1, 2001. 

Boss, Alan P. “Possible Rapid Gas Giant Planet Formation in the Solar Nebula and Other Protoplanetary Disks.” The Astrophysical Journal, 536:L101-104, June 20, 2000. 

Butler, Paul, et al. “Attaining Doppler Precision of 3 m s -1.” Publications of the Astronomical Society of the Pacific, v 108: 500-509, June 1996. 

Butler, Paul. “A precision Velocity Study of Photometrically Stable Stars in the Cepheid Instability Strip.” The Astrophysical Journal, 494: 342-365, February 10, 1998. 

Butler, Paul et al. “Planetary Companions to the Metal-Rich Stars BD -10^o 3166 and HD 52265.” The Astrophysical Journal, 545: 504 – 511, December 10, 2000. 

DARWIN Website: http://www.darwin.rl.ac.uk/index.htm  

Freedman, Roger A. and William J. Kaufmann III. Universe. Seventh Edition. W.H. Freeman and Company, New York. 2005. 

Goldreich, Peter; Lithwick, Yoram and Re’em Sari. “Final Stages of Planet Formation.” The Astrophysical Journal, 614:497-507, October 10, 2004. 

Innanen, Kimmo; Mikkola, Seppo and Paul Wiegert. “The Earth-Moon System and the Dynamical Stability of the Inner Solar System.” The Astrophysical Journal, 116:2055-2057. October 1998. 

Kitchin, C. R. Astrophysical Techniques 3^rd Edition. Institute of Physics Publishing. Bristol, 1998. 

Lunine, Jonathan. ­Astrobiology. A Multidisciplinary Approach. Pearson Addison Wesley, San Francisco. 2005. 

Marcy, G. W. and Paul Butler. “Characteristics of Observed Extrasolar Planets.” The Tenth Cambridge Workshop on Cool Stars, Stellar Systems and the Sun. Cambridge, Massachusetts. July 16-20, 1997. 

Mayor, Michael and Pierre-Yves Frei. New World in the Cosmos. The Discovery of Exoplanets. Cambridge University Press, 2003. 

McDowell, Jonathan. “NASA Science in Free Fall.” Sky and Telescope Magazine, pg 16-17. June 2006. 

Murphy, Ricky. “On the Stability of the Gliese 876 System of Planets and the Importance of the Inner Planet.” HET617 Project, November 2005. Internet. 

Sozzetti, Alessandro. “Astrometric Methods and Instrumentations to Identify and Characterize Extrasolar Planets: A Review.” PASP, 117:1021-1048. October, 2005. 

Tonkin, Stephen. Practical Amateur Spectroscopy. Springer. London, 2003. 

Back to Top

Image Credits: 

[I1] http://oz.plymouth.edu/~sci_ed/Turski/Courses/Earth_Science/Intro.html  

[I2] http://hubblesite.org/newscenter/newsdesk/archive/releases/1995/45/image/b  

[I3] http://hubblesite.org/newscenter/newsdesk/archive/releases/1999/05/image/c  

[I4] http://hubblesite.org/newscenter/newsdesk/archive/releases/2000/02/  

[I5] http://www.eso.org/outreach/press-rel/pr-2005/pr-12-05-pho http://vo.obspm.fr/exoplanetes/encyclo/catalog-RV.php?mdAff=diag#tc  

[I6] Earthshine: http://www.spaceref.com/news/viewpr.html?pid=7055

Back to Top