Introduction
Image Acquisition and Reduction
Calibration
The Color Magnitude Diagram
Analysis
Conclusion
References

Back to Astrophotography

Introduction:

A color magnitude diagram is a variant of the Hertzsprung-Russell diagram. While the Hertzsprung-Russell (H-R) diagram is a summary of temperatures and magnitudes of individual stars, a color magnitude diagram (CMD) is dedicated to the study of star clusters. The two most common star clusters are globular and open. A globular cluster contains thousands of stars and is considered old in comparison to other clusters (Ostlie, page 529). They also tend to organize outside the main disk of a galaxy. Open clusters on the other hand are considered young, and exist within the main disk of a galaxy (Ostlie, page 530). The purpose of this project is to create a CMD of an open cluster, M67, and give a brief analysis of the result. In order to plot this diagram accurately, it is required that the images be calibrated to a standard scale. Images are provided by Pamela Gay from the McDonald Observatory in Davis Texas. In addition to images of M67, standard Landolt fields were imaged, as well as the globular cluster NGC4147. The Landolt fields and NGC4147 will be used to create a calibration scale and the results applied to the images of M67.

Back to Top | Back to Astrophotography

Image Acquisition and Reduction:

Use of spectral filters to acquire an image is standard practice when imaging star fields for photometric analysis; however, every telescope will influence the image with its color term. In an effort to provide a standard, Arlo Landolt has created a system of calibration based on the Johnson-Kron-Cousins photometric system. Using a standard filter set, Professor Landolt cataloged 526 stars along the celestial equator and documented each of these stars through UVBRI filters and averaged the result (Landolt, 1992). These results are considered the standard photometric system to which all other telescopes are to calibrate. The result of this hard work is obvious: no mater the style, type, or size of a telescope, an accurate CMD can be generated.

While the filters used for the Landolt series were UVBRI, our diagram will be extrapolated from BRI images.

The reason for selecting various individual color filter images is to create a degree of magnitude difference between them as an indication of color index – which can be translated to temperature.

Figure 1.

In addition to calibrating the color term introduced by a telescope, the CCD camera used to acquire the images must also be calibrated. While a single image from a CCD camera can be calibrated to true black (using the overscan area), noise and heat induces small changes in levels as more images are acquired. Because of this, an image called a bias frame is required to calibrate every image according to the levels on this one frame. In addition to the bias frame, a flat field must also be captured. By capturing an image with the aperture blocked, noise and artifacts are still acquired. When this image is applied to the other images, the majority of noise and damage to the CCD chip will be subtracted from the image leaving only the desired result. This entire process is called image reduction. MaxImDL is used to calibrate the images, and extract photometric information from the two given Landolt standard fields, NGC4147, and M67. Please see the appendix

Image Reduction – step by step.

In addition to image reduction, it is also necessary to create a photometric plot of each image. The process of photometric extraction is also outlined in the Image Reduction – step by step appendix. By selecting specific stars within the Landolt fields (fields SA104 and SA107 in this case) as well as specific stars indicated by Pamela Gay within NGC4147, photometric information from the provided images are compared to the Landolt standards.

Our subject: M67 in RGB. The green channel is synthetic, thanks to Registar.

Back to Top | Back to Astrophotography

Calibration:

The first step in calibration of all the images is to organize the photometric data extracted by MaxImDL into an Excel spreadsheet. The purpose of calibration is to determine the color term, which is the value of a resulting mathematical expression used to compare photometric results with a list of standard stars provided by the Landolt UVBRI (BRI in our case) Photometric Standard Stars (Landolt, 1992). To solve for the color term, the following equations are programmed into the attached Excel spreadsheet, care of Pamela Gay:

mB = [(B-R) + R] + x₁B + x₂B ´ Airmass + x₃B ´ (B-R),

mR = R + x₁R + x₂R ´ Airmass + x₃R ´ (B-R),

mI = [R – (R – I)] + x₁I + x₂I ´ Airmass + x₃I ´ (R-I)[1].

mB, mR, and mI         =          instrument magnitude

B, R, I                        =          Landolt magnitude

x1                              =          constant

x2                              =          airmass[2]

x3                              =          color term

In order to pinpoint the exact color term of our telescope, we must plot scatter charts within Excel of the B, R, and I images. Since Excel will be used to generate the scatter plots for these three filters, it is easy to constrain the results to a standard deviation of <0.2 and a median of 0 +/- 0.08 in comparison with the calculated Landolt measurements – both are internal functions within the program.

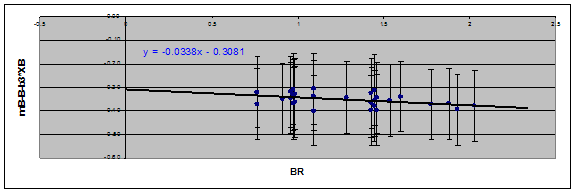
Figure 2.

The plot above gives the slope of instrument magnitudes compared to Landolt measured magnitudes for the B filter.

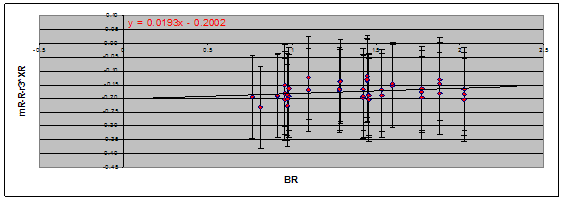
Figure 3.

The above plot is the slope of the R filter images.

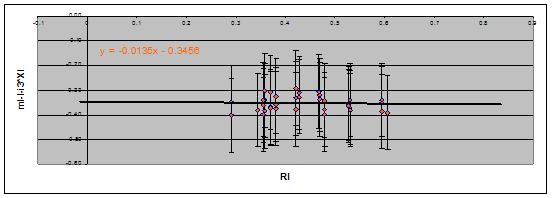
Figure 4.

This plot is the slope from the I filter images.

The resulting plots provides us with the values for the constant (x1) and the color term of each filter (x2) specific to the telescope used to

Figure 5.

capture the provided images.

The three plots above share the same pattern: the horizontal axis is the calculated Landolt values: B-R for the blue and red filters, and R-I for the infrared filter; the vertical axis is the result of our instrument measurements with a constant and the airmass values in comparison to the Landolt values. Specifically the plot for the blue and red filters has the vertical values based on:

                                    mB – B – b3 * XB and mR – R – r3 * XR,

where mB (mR) is the instrument magnitude, B (R) is the Landolt magnitude, XB (XR) is the airmass value, b3 is a constant value of 0.263 and r3 is a constant value of 0.159[3]. The term for the I filter is ignored since our CMD will plot stars based on the B-R color index.

Back to Top | Back to Astrophotography

The Color Magnitude Diagram:

Now that all of the hard work is out of the way, we can now concentrate on creating our very own CMD. With the constants generated by the calibration method, we are now able to use the photometry measurements of star cluster M67 and place it on a standard scale. To make things simple, we will only create a CMD with a color index of B-R. The magnitude of the stars representing the color index will run on the vertical axis while the B-R will run on the horizontal axis.

The attached spreadsheet contains the individual star data as well as the generated CMD. In order to put our plot of M67 to the Landolt standards, two equations are used.

This first equation generates the color term based on the selected stars:

B-R = (mB – mR) – (x1B – x1R) – (0.263 * XB – 0.159 * XR) / (1 + x2B – x2R)

Where x1B = -0.3031 (the constant value from calibration), and x1R = -0.2002.

Once these values are calculated, the standardized apparent magnitudes of the stars are calculated by:

R = (mR – x1R – x2R * BR – 0.159 * XR)

Where x2R = 0.0193 (the color term), and BR is the value from the first equation.

For comparison purposes, let’s take a look at a standard Hertzsprung-Russell (H-R) diagram:

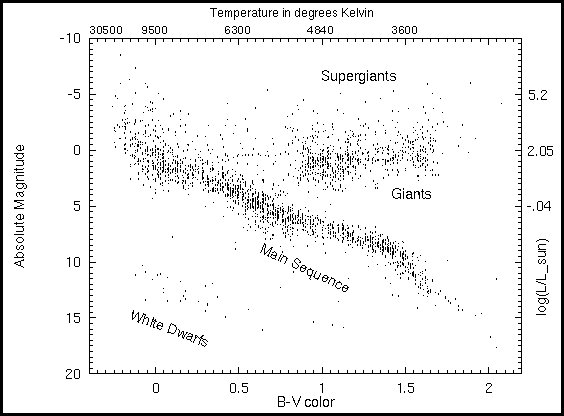
Figure 6. (Image borrowed from: http://www.astronomynotes.com/starprop/s13.htm)

Notice absolute magnitude scale on the right and the B-V color on the bottom. Our CMD will have the same orientation.

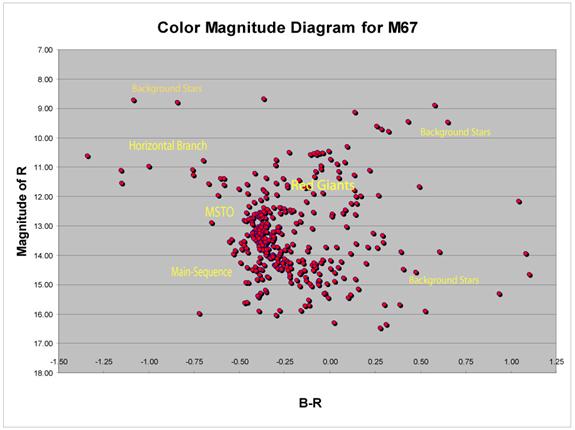
Figure 7.

With a sample of 373 stars, our CMD contains enough information to make out several key features of this diagram. At first glance, it would appear that the resulting graph is a culmination of random stars; however, the concentration of stars near the center has an appearance of a main-sequence belt. With the few background stars ignored, it is also possible to see a group of stars populate the area indicative of the red giant phase on the H-R diagram, as well as a possible horizontal branch near the upper left of the diagram. Of significance is the appearance of a clear cut-off of stars at the tip of the main-sequence. This area refers to the main-sequence cut-off (MSTO) which is higher main-sequence stars that have used up their supply of hydrogen, and are now in a core helium burning stage. Since all the stars in a cluster form around the same time from the same interstellar dust cloud, this cut-off demonstrates that the larger, faster burning stars have already left the main-sequence and are populating the red giant area of the diagram.

Back to Top | Back to Astrophotography

Analysis:

The study of a color magnitude diagram can reveal a host of information about stellar evolution. With only 373 stars plotted on our diagram and only one color index featured, it would be difficult to generate accurate information regarding stellar features such as surface temperature, age, metallicity, and distance; however, we can infer with reasonable certainty that our CMD can constrain these values to an acceptable degree. With a B-R value of 1.03 (Doressoundiram, 2002), our Sun can serve as the focal point so our CMD can be overlaid to a know H-R diagram (Figure 6). Once the reference is made, we can clearly see that our CMD is composed of high mass stars still residing on the main-sequence; while the higher mass stars have successfully entered the red giant phase. It is safe to say that A and B spectral type stars still exist on our main-sequence while the hottest O and OB stars have turned off the main-sequence. With the A and B stars still on the main-sequence, we can estimate this cluster is at least 15 x 10^6 years of age (Freedman, page 481). With the bright B-R values of our plot, we can infer the presence of abundant metals (Chiboucas, Internet) making the stars of our cluster Population I stars. This is in agreement with open clusters being younger in age that globular clusters. To estimate the distance to this cluster, we will use the ever famous distance modulus:

m – M = 5 log d – 5.

Using our CMD as the guide, and inserting the absolute magnitude of a star with a B-V[4] of 0, we know this B type star has an absolute magnitude of -2[5].

d = 10^(m-M+5)/5 pc.

d = 10^(15 – 2 + 5)/5 pc.

d = 3981 pc.

Back to Top | Back to Astrophotography

Conclusion:

By using a standard calibrating system, we were able to calibrate images of star cluster M67. A color magnitude diagram is a type of H-R diagram that is used as a tool in studying a star cluster. Our CMD of M67 was able to reveal some very useful information. We are able to determine that this cluster is metal rich, contains mostly high mass stars, is around 15 x 10^6 years old, and has a distance of about 3900 parsecs. While the information provided is only a rough estimate, it is clear that a CMD has much to tell us. One of the most important aspects of a color magnitude diagram is its ability to help us understand stellar evolution (Ostlie, page 531). It is also possible that CMD’s can provide valuable information to the formation of white-dwarfs, and give insight to a fairly new stellar body called the blue straggler[6]. We have much to learn about stellar evolution, but now we have the tools to help us understand.

Back to Top | Back to Astrophotography

References:

Chiboucas, Kristin. “Research Interests.” http://www.astro.lsa.umich.edu/~kristin/html/interests.html

Doressoundiram, A. Et Al. “The Color Distrobution in the Edgewoth-Kuiper Belt.” The Astrophysical Journal, October 2002.

Freedman, Roger. William Kaufman. Universe: Sixth Edition. W.H. Freeman and Company, New York. 2002.

Landolt, Arlo. “UBVRI Photometric Standard Stars in the Magnitude Range 11.5 < V < 16.0 Around the Celestial Equator.” The Astrophysical Journal, Volume 104, Number 1, July 1992.

Ostlie, Dale. Bradley Carroll. An Introduction to: Modern Stellar Astrophysics. Addison-Wesley Publishing Company, Massachusetts. 1996.

Hourly Airmass Table. http://imagiware.com/astro/airmass.cgi. Internet.

Strobel, Nick. “Astronomy Notes.” www.astronomynotes.com. Internet, 2004.

Back to Top | Back to Astrophotography